A quadrilateral ABCD is drawn to circumscribe a circle (see Fig. 10.12). Prove that

AB + CD = AD + BC

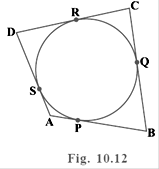
In the given figure, it can be observed that AB touches the circle at point P; BC touches the circle at point Q; CD touches the circle at point R;  DA touches the circle at point S. 

Then, we can say from a theorem that " Length of tangents drawn from an external point to the circle are same ", 
Therefore, 

DR = DS (Tangents on the circle from point D) ….. (1) 

CR = CQ (Tangents on the circle from point C) …... (2)

BP = BQ (Tangents on the circle from point B) ….... (3)

AP = AS (Tangents on the circle from point A) ….... (4)

Adding all these equations, we obtain

DR + CR + BP + AP = DS + CQ + BQ + AS

(DR + CR) + (BP + AP) = (DS + AS) + (CQ + BQ)

= CD + AB = AD + BC Proved.