Given the equation of the ellipse is 9x² + 25y² = 225.

We need to find the eccentricity, coordinates of foci and length of latus rectum.

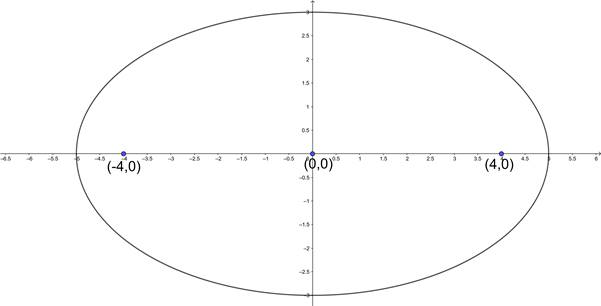
Given equation can be rewritten as

⇒ .

We know for the ellipse (a²>b²)

⇒

⇒ Coordinates of foci (±ae,0)

⇒ Length of latus rectum =

Here a² = 25 and b² = 9, a²>b²

⇒

⇒

⇒

⇒

⇒

⇒ Length of latus rectum (L) =

⇒

∴ The eccentricity is , foci are and length of the latus rectum is .