Table of Contents

Chapter 10
Practical Geometry
10.1 Introduction
You are familiar with a number of shapes. You learnt how to draw some of them in the earlier classes. For example, you can draw a line segment of given length, a line perpendicular to a given line segment, an angle, an angle bisector, a circle etc.
Now, you will learn how to draw parallel lines and some types of triangles.
10.2 Construction of a Line Parallel to a Given Line, Through a Point not on the Line
Let us begin with an activity (Fig 10.1)
(i) Take a sheet of paper. Make a fold. This fold represents a line l.
(ii) Unfold the paper. Mark a point A on the paper outside l.
(iii) Fold the paper perpendicular to the line such that this perpendicular passes through A. Name the perpendicular AN.
(iv) Make a fold perpendicular to this perpendicular through the point A. Name the new perpendicular line as m. Now, l || m. Do you see ‘why’?
Which property or properties of parallel lines can help you here to say that lines l and m are parallel.
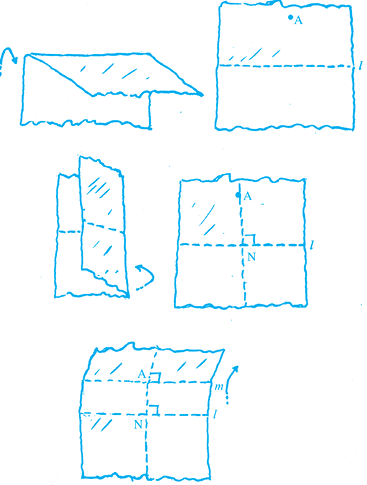
Fig 10.1
You can use any one of the properties regarding the transversal and parallel lines to make this construction using ruler and compasses only.

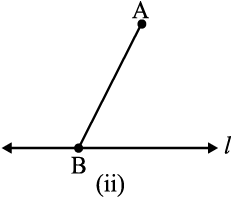
Step 2 Take any point B on l and join B to A [Fig 10.2(ii)].

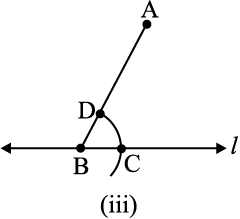
Step 3 With B as centre and a convenient radius, draw an arc cutting l at C and BA at D
[Fig 10.2(iii)].

Step 4 Now with A as centre and the same radius as in Step 3, draw an arc EF cutting AB at G [Fig 10.2 (iv)].
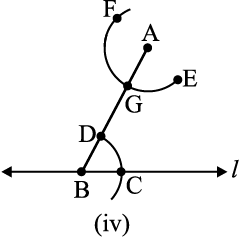
Step 5 Place the pointed tip of the compasses at C and adjust the opening so that the pencil tip is at D [Fig 10.2 (v)].
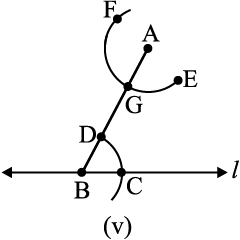
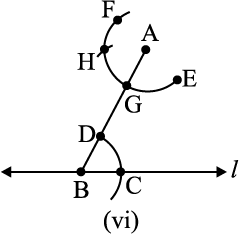

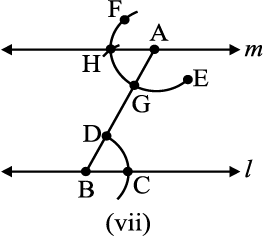
Therefore m || l
Think, Discuss and Write
1. In the above construction, can you draw any other line through A that would be also parallel to the line l?
2. Can you slightly modify the above construction to use the idea of equal corresponding angles instead of equal alternate angles?
Exercise 10.1
1. Draw a line, say AB, take a point C outside it. Through C, draw a line parallel to AB using ruler and compasses only.
2. Draw a line l. Draw a perpendicular to l at any point on l. On this perpendicular choose a point X, 4 cm away from l. Through X, draw a line m parallel to l.
3. Let l be a line and P be a point not on l. Through P, draw a line m parallel to l. Now join P to any point Q on l. Choose any other point R on m. Through R, draw a line parallel to PQ. Let this meet l at S. What shape do the two sets of parallel lines enclose?
10.3 Construction of Triangles
It is better for you to go through this section after recalling ideas on triangles, in particular, the chapters on properties of triangles and congruence of triangles.
You know how triangles are classified based on sides or angles and the following important properties concerning triangles:
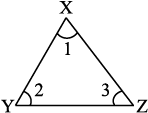
(i) The exterior angle of a triangle is equal in measure to the sum of interior opposite angles.
(ii) The total measure of the three angles of a triangle is 180°.
(iii) Sum of the lengths of any two sides of a triangle is greater than the length of the third side.
(iv) In any right-angled triangle, the square of the length of hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
∠3 = ∠1 + ∠2

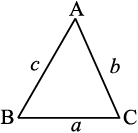
a+ b > c
∠1 + ∠2 + ∠3 = 180°
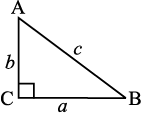
b2+ a2 = c2
In the chapter on ‘Congruence of Triangles’, we saw that a triangle can be drawn if any one of the following sets of measurements are given:
(i) Three sides.
(ii) Two sides and the angle between them.
(iii) Two angles and the side between them.
(iv) The hypotenuse and a leg in the case of a right-angled triangle.
We will now attempt to use these ideas to construct triangles.
10.4 Constructing a Triangle when the Lengths of its Three Sides are Known (SSS Criterion)
In this section, we would construct triangles when all its sides are known. We draw first a rough sketch to give an idea of where the sides are and then begin by drawing any one of
the three lines. See the following example:
Example 1 Construct a triangle ABC, given that AB = 5 cm, BC = 6 cm and AC = 7 cm.
Solution
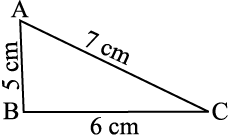
(i)
Step 2 Draw a line segment BC of length 6 cm [Fig 10.3(ii)].
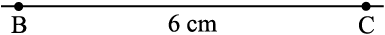
(ii)
Step 3 From B, point A is at a distance of 5 cm. So, with B as centre, draw an arc of radius 5 cm. (Now A will be somewhere on this arc. Our job is to find where exactly A is) [Fig 10.3(iii)].

(iii)
Step 4 From C, point A is at a distance of 7 cm. So, with C as centre, draw an arc of radius 7 cm. (A will be somewhere on this arc, we have to fix it) [Fig 10.3(iv)].
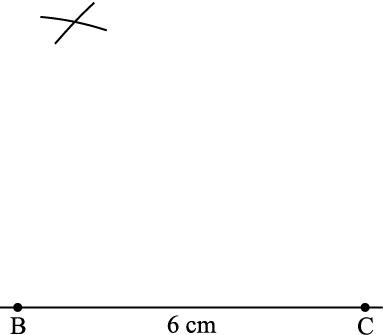
(iv)
Step 5 A has to be on both the arcs drawn. So, it is the point of intersection of arcs.
Mark the point of intersection of arcs as A. Join AB and AC. ∆ABC is now ready [Fig 10.3(v)].

(v)
Fig 10.3 (i)–(v)
Do This
We observe that ∆DEF exactly coincides with ∆ABC. (Note that the triangles have been constructed when their three sides are given.) Thus, if three sides of one triangle are equal to the corresponding three sides of another triangle, then the two triangles are congruent. This is SSS congruency rule which we have learnt in our earlier chapter.
Think, Discuss and Write
A student attempted to draw a triangle whose rough figure is given here. He drew QR first. Then with Q as centre, he drew an arc of 3 cm and with R as centre, he drew an arc of
2 cm. But he could not get P. What is the reason? What property of triangle do you know in connection with this problem?
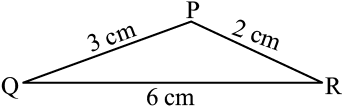
Fig 10.4 Think: Is this right?
Can such a triangle exist? (Remember the property of triangles ‘The sum of any two sides of a traingle is always greater than the third side’!)
Exercise 10.2
1. Construct ∆xyz in which xy = 4.5 cm, yz = 5 cm and zx = 6 cm.
2. Construct an equilateral triangle of side 5.5 cm.
3. Draw ∆PQR with PQ = 4 cm, QR = 3.5 cm and PR = 4 cm. What type of triangle is this?
4. Construct ∆ABC such that AB = 2.5 cm, BC = 6 cm and AC = 6.5 cm. Measure ∠B.

10.5 Constructing a Triangle when the Lengths of Two Sides and the Measure of the Angle Between them are Known. (SAS Criterion)
Here, we have two sides given and the one angle between them. We first draw a sketch and then draw one of the given line segments. The other steps follow. See Example 2.
Example 2 Construct a triangle PQR, given that PQ = 3 cm, QR = 5.5 cm and ∠PQR = 60°.
Solution
Step 1 First, we draw a rough sketch with given measures. (This helps us
to determine the procedure in construction) [Fig 10.5(i)].
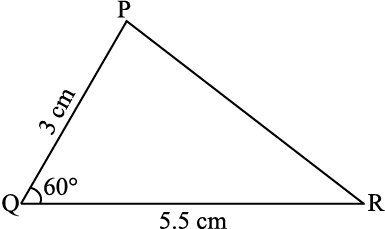
(i)
(Rough Sketch)
Step 2 Draw a line segment QR of length
5.5 cm [Fig 10.5(ii)].
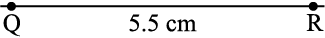
(ii)
Step 3 At Q, draw QX making 60° with QR.
(The point P must be somewhere
on this ray of the angle)
[Fig 10.5(iii)].
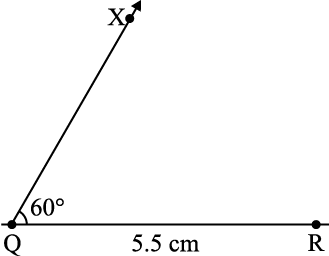
(iii)
Step 4 (To fix P, the distance QP has been given).
With Q as centre, draw an arc of radius 3 cm. It cuts QX at the point P
[Fig 10.5(iv)].

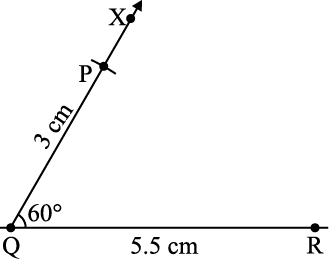
(iv)
Step 5 Join PR. ∆PQR is now obtained (Fig 10.5(v)).
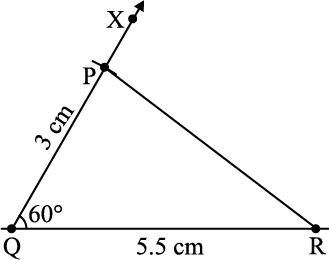
(v)
![]() Do This
Do This

Think, Discuss and Write
In the above construction, lengths of two sides and measure of one angle were given. Now study the following problems:
In ∆ABC, if AB = 3cm, AC = 5 cm and m∠C = 30°. Can we draw this triangle? We may draw AC = 5 cm and draw ∠C of measure 30°. CA is one arm of ∠C. Point B should be lying on the other arm of ∠C. But, observe that point B cannot be located uniquely. Therefore, the given data is not sufficient for construction of ∆ABC.
Now, try to construct ∆ABC if AB = 3cm, AC = 5 cm and m∠B = 30°. What do we observe? Again, ∆ABC cannot be constructed uniquely. Thus, we can conclude that a unique triangle can be constructed only if the lengths of its two sides and the measure of the included angle between them is given.
Exercise 10.3
1. Construct ∆DEF such that DE = 5 cm, DF = 3 cm and m∠EDF = 90°.
2. Construct an isosceles triangle in which the lengths of each of its equal sides is 6.5 cm and the angle between them is 110°.
3. Construct ∆ABC with BC = 7.5 cm, AC = 5 cm and m∠C = 60°.
10.6 Constructing a Triangle when the Measures of two of its Angles and the Length of the Side included between Them is Given. (ASA Criterion)
As before, draw a rough sketch. Now, draw the given line segment. Make angles on the two ends. See the Example 3.
Example 3 Construct ∆XYZ if it is given that XY = 6 cm, m∠ZXY = 30° and m∠XYZ = 100°.
Solution
Step 1 Before actual construction, we draw a rough sketch with measures marked on it. (This is just to get an idea as how to proceed)
[Fig 10.6(i)].
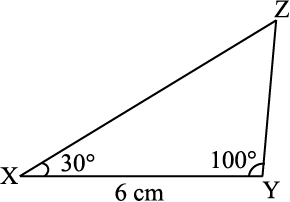
(Rough Sketch)
(i)
Step 2 Draw xy of length 6 cm.

(ii)
Step 3 At x, draw a ray XP making an angle of 30° with xy. By the given condition z must be somewhere on the xp.


(iii)
Step 4 At y, draw a ray yq making an angle of 100° with yx. By the given condition, z must be on the ray yq also.
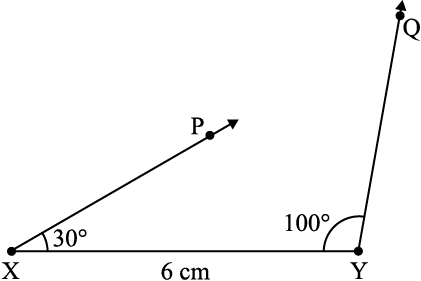
(iv)
Step 5 z has to lie on both the rays xp and yq. So, the point of intersection of the two rays is z.
∆xyz is now completed.
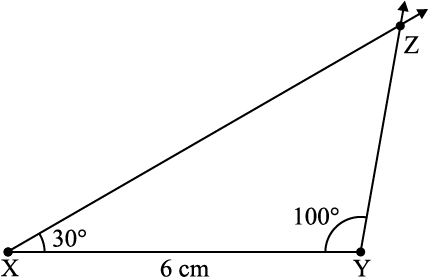
![]() Do This
Do This
Now, draw another ∆LMN, where m∠NLM = 30°, LM = 6 cm and m∠NML = 100°. Take a cutout of ∆LMN and place it on the ∆xyz. We observe that ∆LMN exactly coincides with ∆xyz. Thus, if two angles and the included side of one triangle are equal to the corresponding two angles and the included side of another triangle, then the two triangles are congruent. This is ASA congruency rule which you have learnt in the earlier chapter. (Note that the triangles have been constructed when two angles and the included side between these angles are given.)
Think, Discuss and Write
In the above example, length of a side and measures of two angles were given. Now study the following problem:
In ∆ABC, if AC = 7 cm, m∠A = 60° and m∠B = 50°, can you draw the triangle? (Angle-sum property of a triangle may help you!)
Exercise 10.4
1. Construct ∆ABC, given m∠A = 60°, m∠B = 30° and AB = 5.8 cm.
2. Construct ∆PQR if PQ = 5 cm, m∠PQR = 105° and m∠QRP = 40°.
(Hint: Recall angle-sum property of a triangle).
3. Examine whether you can construct ∆DEF such that EF = 7.2 cm, m∠E = 110° and m∠F = 80°. Justify your answer.
10.7 Constructing a Right-Angled Triangle when the Length of One Leg and its Hypotenuse are Given (RHS Criterion)
Here it is easy to make the rough sketch. Now, draw a line as per the given side. Make a right angle on one of its points. Use compasses to mark length of side and hypotenuse of the triangle. Complete the triangle. Consider the following:
Example 4 Construct ∆LMN, right-angled at M, given that LN = 5 cm and MN = 3 cm.
Solution
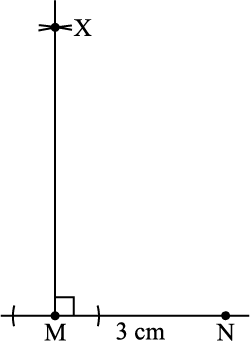
Step 1 Draw a rough sketch and mark the measures. Remember to mark the right angle [Fig 10.7(i)].

(Rough Sketch)
(i)
Step 2 Draw MN of length 3 cm.
[Fig 10.7(ii)].

(ii)
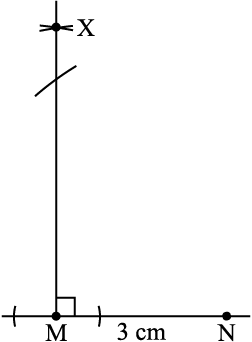
(iii)
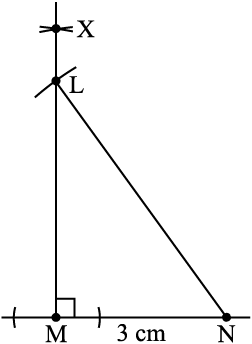
Step 4 With N as centre, draw an arc of radius 5 cm.
(L must be on this arc, since it is at a distance of 5 cm from N) [Fig 10.7(iv)].
(iv)
Step 5 L has to be on the perpendicular line MX as well as on the arc drawn with centre N. Therefore, L is the meeting point of these two.
∆LMN is now obtained.
[Fig 10.7 (v)]

(v)
Exercise 10.5
1. Construct the right angled ∆PQR, where m∠Q = 90°, QR = 8cm and
PR = 10 cm.
2. Construct a right-angled triangle whose hypotenuse is 6 cm long and one of the legs is 4 cm long.
3. Construct an isosceles right-angled triangle ABC, where m∠ACB = 90° and AC = 6 cm.
Miscellaneous questions
Below are given the measures of certain sides and angles of triangles. Identify those which cannot be constructed and, say why you cannot construct them. Construct rest of the triangles.
Triangle Given measurements
1. ∆ABC m∠A = 85°; m∠B = 115°; AB = 5 cm.
2. ∆PQR m∠Q = 30°; m∠R = 60°; QR = 4.7 cm.
3. ∆ABC m∠A = 70°; m∠B = 50°; AC = 3 cm.
4. ∆LMN m∠L = 60°; m∠N = 120°; LM = 5 cm.
5. ∆ABC BC = 2 cm; AB = 4 cm; AC = 2 cm.
6. ∆PQR PQ = 3.5 cm.; QR = 4 cm.; PR = 3.5 cm.
7. ∆xyz xy = 3 cm; yz = 4 cm; xz = 5 cm
8. ∆DEF DE = 4.5cm; EF = 5.5cm; DF = 4 cm.
What have we Discussed?
In this Chapter, we looked into the methods of some ruler and compasses constructions.
We could also have used the idea of ‘equal corresponding angles’ to do the construction.
2. We studied the method of drawing a triangle, using indirectly the concept of congruence of triangles.
The following cases were discussed:
(i) SSS: Given the three side lengths of a triangle.
(ii) SAS: Given the lengths of any two sides and the measure of the angle between these sides.
(iii) ASA: Given the measures of two angles and the length of side included between them.
(iv) RHS: Given the length of hypotenuse of a right-angled triangle and the length of one of its legs.


 D
D