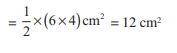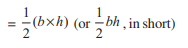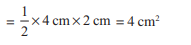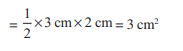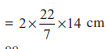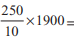Table of Contents
Chapter 11
Perimeter and Area
11.1 Introduction
In Class VI, you have already learnt perimeters of plane figures and areas of squares and rectangles. Perimeter is the distance around a closed figure while area is the part of plane or region occupied by the closed figure.
In this class, you will learn about perimeters and areas of a few more plane figures.
11.2 Squares and Rectangles
Ayush and Deeksha made pictures. Ayush made his picture on a rectangular sheet of length 60 cm and breadth 20 cm while Deeksha made hers on a rectangular sheet of length 40 cm and breadth 35 cm. Both these pictures have to be separately framed and laminated.
Who has to pay more for framing, if the cost of framing is ₹ 3.00 per cm?
If the cost of lamination is ₹ 2.00 per cm2, who has to pay more for lamination?
For finding the cost of framing, we need to find perimeter and then multiply it by the rate for framing. For finding the cost of lamination, we need to find area and then multiply it by the rate for lamination.
Try These
What would you need to find, area or perimeter, to answer the following?
1. How much space does a blackboard occupy?
2. What is the length of a wire required to fence a rectangular flower bed?
3. What distance would you cover by taking two rounds of a triangular park?
4. How much plastic sheet do you need to cover a rectangular swimming pool?
Do you remember,
Perimeter of a regular polygon = number of sides × length of one side
Perimeter of a square = 4 × side
Perimeter of a rectangle = 2 × (l + b)
Area of a rectangle = l × b, Area of a square = side × side
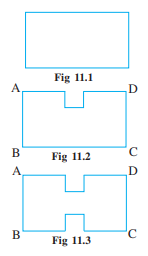
Tanya needed a square of side 4 cm for completing a collage. She had a rectangular sheet of length 28 cm and breadth 21 cm (Fig 11. 1). She cuts off a square of side 4 cm from the rectangular sheet. Her friend saw the remaining sheet (Fig 11.2) and asked Tanya, “Has the perimeter of the sheet increased or decreased now?”
Has the total length of side AD increased after cutting off the square?
Has the area increased or decreased?
Tanya cuts off one more square from the opposite side (Fig 11.3).
Will the perimeter of the remaining sheet increase further?
Will the area increase or decrease further?
So, what can we infer from this?
It is clear that the increase of perimeter need not lead to increase in area.
Try These
1. Experiment with several such shapes and cut-outs. You might find it useful to draw these shapes on squred sheets and compute their areas and perimeters.
You have seen that increase in perimeter does not mean that area will also increase.
2. Give two examples where the area increases as the perimeter increases.
3. Give two examples where the area does not increase when perimeter increases.
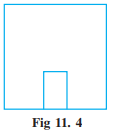
Example 1 A door-frame of dimensions 3 m × 2 m is fixed on the wall of dimension 10 m × 10 m. Find the total labour charges for painting the wall if the labour charges for painting 1 m2 of the wall is ₹ 2.50.
Solution Painting of the wall has to be done excluding the area of the door.
Area of the door = l × b
= 3 × 2 m2 = 6 m2
Area of wall including door = side × side = 10 m × 10 m = 100 m2
Area of wall excluding door = (100 − 6) m2 = 94 m2
Total labour charges for painting the wall = ₹ 2.50 × 94 = ₹ 235
Example 2 The area of a rectangular sheet is 500 cm2. If the length of the sheet is 25 cm, what is its width? Also find the perimeter of the rectangular sheet.
Solution Area of the rectangular sheet = 500 cm2
Length (l) = 25 cm
Area of the rectangle = l × b (where b = width of the sheet)
Therefore, width b = 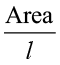 =
= 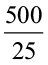 = 20 cm
= 20 cm
Perimeter of sheet = 2 × (l + b) = 2 × (25 + 20) cm = 90 cm
So, the width of the rectangular sheet is 20 cm and its perimeter is 90 cm.
Example 3 Anu wants to fence the garden in front of her house (Fig 11.5), on three sides with lengths 20 m, 12 m and 12 m. Find the

cost of fencing at the rate of 150 per metre.
Solution The length of the fence required is the perimeter of the garden (excluding one side) which is equal to 20 m + 12 m + 12 m, i.e., 44 m.
Cost of fencing = ₹ 150 × 44 = ₹ 6,600.
Example 4 A wire is in the shape of a square of side 10 cm. If the wire is rebent into a rectangle of length 12 cm, find its breadth. Which encloses more area, the square or the rectangle?
Solution Side of the square = 10 cm
Length of the wire = Perimeter of the square = 4 × side = 4 × 10 cm
= 40 cm
Length of the rectangle, l = 12 cm. Let b be the breadth of the rectangle.
Perimeter of rectangle = Length of wire = 40 cm
Perimeter of the rectangle = 2 (l + b)
Thus, 40 = 2 (12 + b)
or 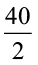 = 12 + b
= 12 + b
Therefore, b = 20 − 12 = 8 cm
The breadth of the rectangle is 8 cm.
Area of the square = (side)2
= 10 cm × 10 cm = 100 cm2
Area of the rectangle = l × b
= 12 cm × 8 cm = 96 cm2
So, the square encloses more area even though its perimeter is the same as that of the rectangle.
Example 5 The area of a square and a rectangle are equal. If the side of the square is 40 cm and the breadth of the rectangle is 25 cm, find the length of the rectangle. Also, find the perimeter of the rectangle.
Solution Area of square = (side)2
= 40 cm × 40 cm = 1600 cm2
It is given that,
The area of the rectangle = The area of the square
Area of the rectangle = 1600 cm2, breadth of the rectangle = 25 cm.
Area of the rectangle = l × b
or 1600 = l × 25
or 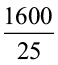 = l or l = 64 cm
= l or l = 64 cm
So, the length of rectangle is 64 cm.
Perimeter of the rectangle = 2 (l + b) = 2 (64 + 25) cm
= 2 × 89 cm = 178 cm
So, the perimeter of the rectangle is 178 cm even though its area is the same as that of the square.
Exercise 11.1
1. The length and the breadth of a rectangular piece of land are 500 m and 300 m respectively. Find
(i) its area (ii) the cost of the land, if 1 m2 of the land costs ₹ 10,000.
2. Find the area of a square park whose perimeter is 320 m.
3. Find the breadth of a rectangular plot of land, if its area is 440 m2 and the length is
22 m. Also find its perimeter.
4. The perimeter of a rectangular sheet is 100 cm. If the length is 35 cm, find its breadth. Also find the area.
5. The area of a square park is the same as of a rectangular park. If the side of the square park is 60 m and the length of the rectangular park is 90 m, find the breadth of the rectangular park.

Fig 11.6
6. A wire is in the shape of a rectangle. Its length is 40 cm and breadth is 22 cm. If the same wire is rebent in the shape of a square, what will be the measure of each side. Also find which shape encloses more area?
7. The perimeter of a rectangle is 130 cm. If the breadth of the rectangle is 30 cm, find its length. Also find the area of the rectangle.
8. A door of length 2 m and breadth 1m is fitted in a wall. The length of the wall is 4.5 m and the breadth is 3.6 m (Fig11.6). Find the cost of white washing the wall, if the rate of white washing the wall is ₹ 20 per m2.
11.2.1 Triangles as Parts of Rectangles
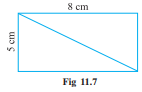
Take a rectangle of sides 8 cm and 5 cm. Cut the rectangle along its diagonal to get two triangles (Fig 11.7).
Superpose one triangle on the other.
Are they exactly the same in size?
Can you say that both the triangles are equal in area?
Are the triangles congruent also?
What is the area of each of these triangles?
You will find that sum of the areas of the two triangles is the same as the area of the rectangle. Both the triangles are equal in area.
The area of each triangle = 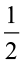 (Area of the rectangle)
(Area of the rectangle)

= 
Take a square of side 5 cm and divide it into 4 triangles as shown (Fig 11.8).
Are the four triangles equal in area?
Are they congruent to each other? (Superpose the triangles to check).
What is the area of each triangle?
The area of each triangle = 
= 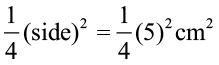 = 6.25 cm2
= 6.25 cm2
11.2.2 Generalising for other Congruent Parts of Rectangles
A rectangle of length 6 cm and breadth 4 cm is divided into two parts as shown in the Fig 11.9. Trace the rectangle on another paper and cut off the rectangle along EF to divide it into two parts.
Superpose one part on the other, see if they match. (You may have to rotate them).
Are they congurent? The two parts are congruent to each other. So, the area of one part is equal to the area of the other part.
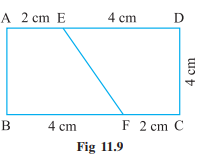
Therefore, the area of
each congruent part = 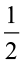 (The area of the rectangle)
(The area of the rectangle)
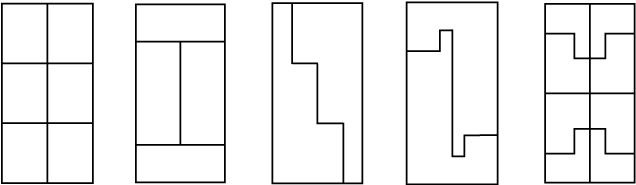
Try These
Each of the following rectangles of length 6 cm and breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
11.3 Area of a Parallelogram
We come across many shapes other than squares and rectangles.
How will you find the area of a land which is a parallelogram in shape?
Let us find a method to get the area of a parallelogram.
Can a parallelogram be converted into a rectangle of equal area?
Draw a parallelogram on a graph paper as shown in Fig 11.10(i). Cut out the parallelogram. Draw a line from one vertex of the parallelogram perpendicular to the opposite side [Fig 11.10(ii)]. Cut out the triangle. Move the triangle to the other side of the parallelogram.
What shape do you get? You get a rectangle.
Is the area of the parallelogram equal to the area of the rectangle formed?
Yes, area of the parallelogram = area of the rectangle formed
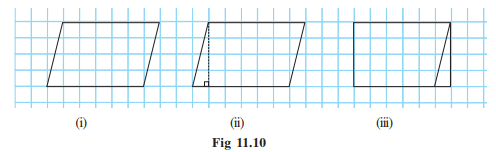
What are the length and the breadth of the rectangle?
We find that the length of the rectangle formed is equal to the base of the parallelogram and the breadth of the rectangle is equal to the height of the parallelogram (Fig 11.11).
Now, Area of parallelogram = Area of rectangle
= length × breadth = l × b
But the length l and breadth b of the rectangle are exactly the base b and the height h, respectively of the parallelogram.
Thus, the area of parallelogram = base × height = b × h.
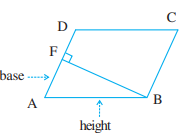
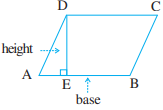
Any side of a parallelogram can be chosen as base of the parallelogram. The perpendicular dropped on that side from the opposite vertex is known as height (altitude). In the parallelogram ABCD, DE is perpendicular to AB. Here AB is the base and DE is the height of the parallelogram.
In this parallelogram ABCD, BF is the perpendicular to opposite side AD. Here AD is the base and BF is the height.
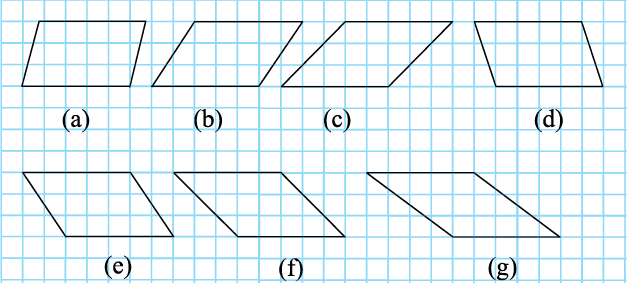
Fig 11.12
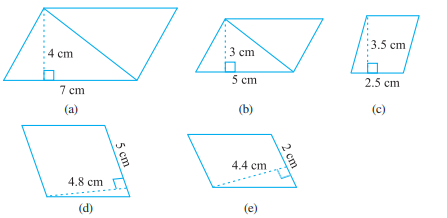
Find the areas of the parallelograms by counting the squares enclosed within the figures and also find the perimeters by measuring the sides.
Complete the following table:
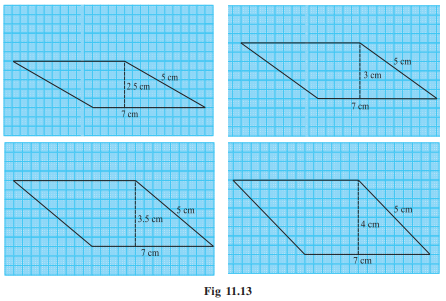
You will find that all these parallelograms have equal areas but different perimeters. Now, consider the following parallelograms with sides 7 cm and 5 cm (Fig 11.13).
Find the perimeter and area of each of these parallelograms. Analyse your results.
You will find that these parallelograms have different areas but equal perimeters.
To find the area of a parallelogram, you need to know only the base and the corresponding height of the parallelogram.
11.4 Area of a Triangle
A gardener wants to know the cost of covering the whole of a triangular garden with grass.
 In this case we need to know the area of the triangular region.
In this case we need to know the area of the triangular region.
Let us find a method to get the area of a triangle.
Draw a scalene triangle on a piece of paper. Cut out the triangle.
Place this triangle on another piece of paper and cut out another triangle of the same size.
So now you have two scalene triangles of the same size.
Are both the triangles congruent?
Superpose one triangle on the other so that they match. You may have to rotate one of the two triangles.
Now place both the triangles such that a pair of corresponding sides is joined as shown in Fig 11.14.
Is the figure thus formed a parallelogram?
Compare the area of each triangle to the area of the parallelogram.
Compare the base and height of the triangles with the base and height of the parallelogram.
You will find that the sum of the areas of both the triangles is equal to the area of the parallelogram. The base and the height of the triangle are the same as the base and the height of the parallelogram, respectively.
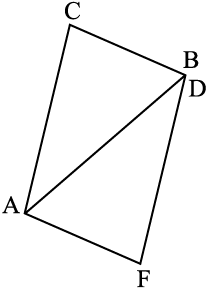
Area of each triangle = 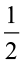 (Area of parallelogram)
(Area of parallelogram)
= 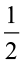 (base × height) (Since area of a parallelogram = base × height)
(base × height) (Since area of a parallelogram = base × height)
TRY THESE
1. Try the above activity with different types of triangles.
2. Take different parallelograms. Divide each of the parallelograms into two triangles by cutting along any of its diagonals. Are the triangles congruent?
In the figure (Fig 11.15) all the triangles are on the base AB = 6 cm.
What can you say about the height of each of the triangles corresponding to the base AB?
Can we say all the triangles are equal in area? Yes.
Are the triangles congruent also? No.
We conclude that all the congruent triangles are equal in area but the triangles equal in area need not be congruent.
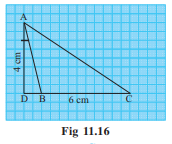
Consider the obtuse-angled triangle ABC of base 6 cm (Fig 11.16).
Its height AD which is perpendicular from the vertex A is outside the triangle.
Can you find the area of the triangle?
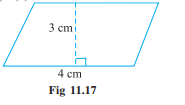
Example 6 One of the sides and the corresponding height of a parallelogram are 4 cm and 3 cm respectively. Find the area of the parallelogram (Fig 11.17).
Solution Given that length of base (b) = 4 cm, height (h) = 3 cm
Area of the parallelogram = b × h
= 4 cm × 3 cm = 12 cm2
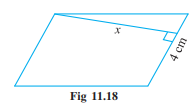
Example 7 Find the height ‘x’ if the area of the parallelogram is 24 cm2 and the base is 4 cm.
Solution Area of parallelogram = b × h
Therefore, 24 = 4 × x (Fig 11.18)
or 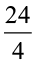 = x or x = 6 cm
= x or x = 6 cm
So, the height of the parallelogram is 6 cm.
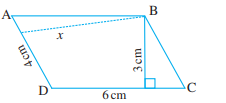
Example 8 The two sides of the parallelogram ABCD are 6 cm and 4 cm. The height corresponding to the base CD is 3 cm (Fig 11.19). Find the
(i) area of the parallelogram. (ii) the height corresponding to the base AD.
Solution
Fig 11.19
(i) Area of parallelogram = b × h
= 6 cm × 3 cm = 18 cm2
(ii) base (b) = 4 cm, height = x (say),
Area = 18 cm2
Area of parallelogram = b × x
18 = 4 × x
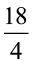 = x
= x
Therefore, x = 4.5 cm
Thus, the height corresponding to base AD is 4.5 cm.
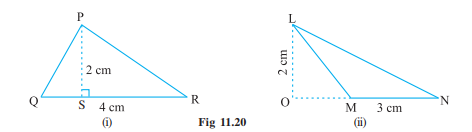
Example 9 Find the area of the following triangles (Fig 11.20).
Solution
(i) Area of triangle = 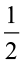 bh =
bh = 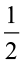 × QR × PS
× QR × PS
(ii) Area of triangle = 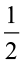 bh =
bh = 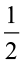 × MN × LO
× MN × LO
Example 10 Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm (Fig 11.21).
Solution Height = 3 cm, Area = 36 cm2
Area of the triangle ABC = 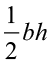
or 36 =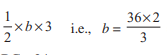
So, BC = 24 cm
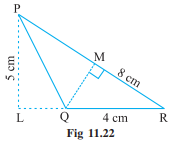
Example 11 In ∆PQR, PR = 8 cm, QR = 4 cm and PL = 5 cm (Fig 11.22). Find:
(i) the area of the ∆PQR (ii) QM
Solution
(i) QR = base = 4 cm, PL = height = 5 cm
Area of the triangle PQR = 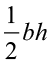
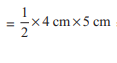
= 10 cm2
(ii) PR = base = 8 cm QM = height = ? Area = 10 cm2
Area of triangle =
h =  =
= 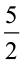 = 2.5. So, QM = 2.5 cm
= 2.5. So, QM = 2.5 cm
Exercise 11.2
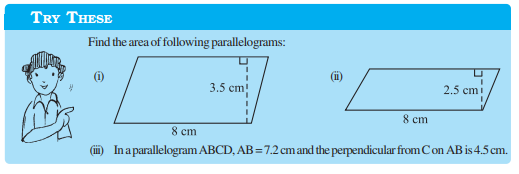
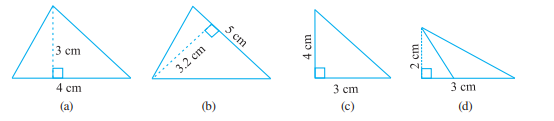
1. Find the area of each of the following parallelograms:
2. Find the area of each of the following triangles:
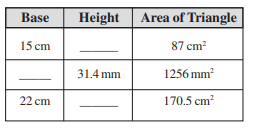
3. Find the missing values:
4. Find the missing values:
5. PQRS is a parallelogram (Fig 11.23). QM is the height from Q to SR and QN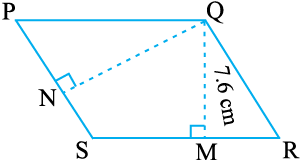 is the height from Q to PS. If SR = 12 cm and QM = 7.6 cm. Find:
is the height from Q to PS. If SR = 12 cm and QM = 7.6 cm. Find:
(a) the area of the parallegram PQRS (b) QN, if PS = 8 cm
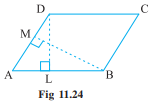
6. DL and BM are the heights on sides AB and AD respectively of parallelogram ABCD (Fig 11.24). If the area of the parallelogram is 1470 cm2, AB = 35 cm and AD = 49 cm, find the length of BM and DL.
7. ∆ABC is right angled at A (Fig 11.25). AD is perpendicular to BC. If AB = 5 cm,
BC = 13 cm and AC = 12 cm, Find the area of ∆ABC. Also find the length of
AD.
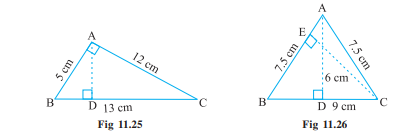
8. ∆ABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm (Fig 11.26). The height AD from A to BC, is 6 cm. Find the area of ∆ABC. What will be the height from C to AB i.e., CE?
11.5 Circles
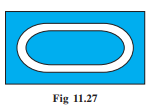
A racing track is semi-circular at both ends (Fig 11.27).
Can you find the distance covered by an athlete if he takes two rounds of a racing track? We need to find a method to find the distances around when a shape is circular.
11.5.1 Circumference of a Circle
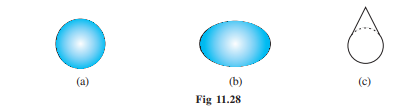
Tanya cut different cards, in curved shape from a cardboard. She wants to put lace around to decorate these cards. What length of the lace does she require for each? (Fig 11.28)
You cannot measure the curves with the help of a ruler, as these figures are not “straight”. What can you do?
Here is a way to find the length of lace required for shape in Fig 11.28(a). Mark a point on the edge of the card and place the card on the table. Mark the position of the point on the table also .
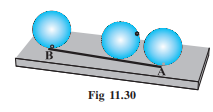
Now roll the circular card on the table along a straight line till the marked point again touches the table. Measure the distance along the line. This is the length of the lace required
(Fig 11.30). It is also the distance along the edge of the card from the marked point back to the marked point.
You can also find the distance by putting a string on the edge of the circular object and taking all round it.
The distance around a circular region is known as its circumference.
Do This
Take a bottle cap, a bangle or any other circular object and find the circumference.
Now, can you find the distance covered by the athlete on the track by this method?
Still, it will be very difficult to find the distance around the track or any other circular object by measuring through string. Moreover, the measurement will not be accurate.
So, we need some formula for this, as we have for rectilinear figures or shapes.
Let us see if there is any relationship between the diameter and the circumference of the circles.
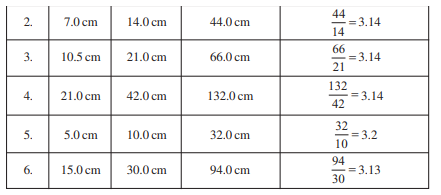
Consider the following table: Draw six circles of different radii and find their circumference by using string. Also find the ratio of the circumference to the diameter.
Circle Radius Diameter Circumference Ratio of Circumference to Diameter
What do you infer from the above table? Is this ratio approximately the same? Yes.
Can you say that the circumference of a circle is always more than three times its diameter? Yes.
This ratio is a constant and is denoted by π (pi). Its approximate value is 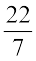 or 3.14.
or 3.14.
So, we can say that 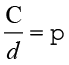 , where ‘C’ represents circumference of the circle and ‘d’ its diameter.
, where ‘C’ represents circumference of the circle and ‘d’ its diameter.
or C = πd
We know that diameter (d) of a circle is twice the radius (r) i.e., d = 2r
So, C = πd = π × 2r or C = 2πr.
Do This
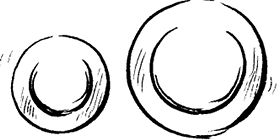 these on a table-top. Which plate covers more distance in one complete revolution? Which plate will take less number of revolutions to cover the length of the table-top?
these on a table-top. Which plate covers more distance in one complete revolution? Which plate will take less number of revolutions to cover the length of the table-top?Example 12 What is the circumference of a circle of diameter 10 cm (Take π = 3.14)?
Solution Diameter of the circle (d) = 10 cm
Circumference of circle = πd
= 3.14 × 10 cm = 31.4 cm
So, the circumference of the circle of diameter 10 cm is 31.4 cm.
Example 13 What is the circumference of a circular disc of radius 14 cm?
Solution Radius of circular disc (r) = 14 cm
Circumference of disc = 2πr
= 88 cm
So, the circumference of the circular disc is 88 cm.
Example 14 The radius of a circular pipe is 10 cm. What length of a tape is required to wrap once around the pipe (π = 3.14)?
Solution Radius of the pipe (r) = 10 cm
Length of tape required is equal to the circumference of the pipe.
Circumference of the pipe = 2πr
= 2 × 3.14 × 10 cm
= 62.8 cm
Therefore, length of the tape needed to wrap once around the pipe is 62.8 cm.
Example 15 Find the perimeter of the given shape (Fig 11.32) (Take π = 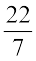 ).
).
Solution In this shape we need to find the circumference of semicircles on each side of the square. Do you need to find the perimeter of the square also? No. The outer boundary, of this figure is made up of semicircles. Diameter of each semicircle is 14 cm.
We know that:
Circumference of the circle = πd
Circumference of the semicircle = 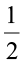 πd
πd
= 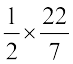 × 14 cm = 22 cm
× 14 cm = 22 cm
Circumference of each of the semicircles is 22 cm
Therefore, perimeter of the given figure = 4 × 22 cm = 88 cm
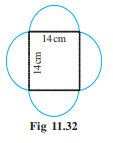
Example 16 Sudhanshu divides a circular disc of radius 7 cm in two equal parts. What is the perimeter of each semicircular shape disc? (Use π = 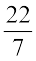 )
)
Solution To find the perimeter of the semicircular disc (Fig 11.33), we need to find
(i) Circumference of semicircular shape (ii) Diameter
Given that radius (r) = 7 cm. We know that the circumference of circle = 2πr
So, the circumference of the semicircle = 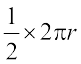 = πr
= πr
= 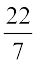 × 7 cm = 22 cm
× 7 cm = 22 cm
So, the diameter of the circle = 2r = 2 × 7 cm = 14 cm
Thus, perimeter of each semicircular disc = 22 cm + 14 cm = 36 cm
11.5.2 Area of Circle
Consider the following:
- A farmer dug a flower bed of radius 7 m at the centre of a field. He needs to purchase fertiliser. If 1 kg of fertiliser is required for 1 square metre area, how much fertiliser should he purchase?
- What will be the cost of polishing a circular table-top of radius 2 m at the rate of ₹ 10 per square metre?
Can you tell what we need to find in such cases, Area or Perimeter? In such cases we need to find the area of the circular region. Let us find the area of a circle, using graph paper.
Draw a circle of radius 4 cm on a graph paper (Fig 11.34). Find the area by counting the number of squares enclosed.
As the edges are not straight, we get a rough estimate of the area of circle by this method.
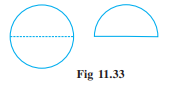
There is another way of finding the area of a circle.
Draw a circle and shade one half of the circle [Fig 11.35(i)]. Now fold the circle into eighths and cut along the folds [Fig 11.35(ii)].
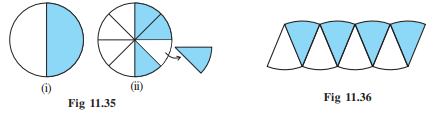
Arrange the separate pieces as shown, in Fig 11.36, which is roughly a parallelogram.
The more sectors we have, the nearer we reach an appropriate parallelogram.
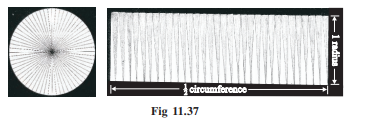
As done above if we divide the circle in 64 sectors, and arrange these sectors. It gives nearly a rectangle (Fig 11.37).
What is the breadth of this rectangle? The breadth of this rectangle is the radius of the circle, i.e., ‘r’.
As the whole circle is divided into 64 sectors and on each side we have 32 sectors, the length of the rectangle is the length of the 32 sectors, which is half of the circumference. (Fig 11.37)
Area of the circle = Area of rectangle thus formed = l × b
= (Half of circumference) × radius = 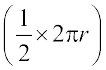 × r = πr2
× r = πr2
So, the area of the circle = πr2
Try These
Draw circles of different radii on a graph paper. Find the area by counting the number of squares. Also find the area by using the formula. Compare the two answers.
Example 17 Find the area of a circle of radius 30 cm (use π = 3.14).
Solution Radius, r = 30 cm
Area of the circle = πr2 = 3.14 × 302 = 2,826 cm2
Example 18 Diameter of a circular garden is 9.8 m. Find its area.
Solution Diameter, d = 9.8 m. Therefore, radius r = 9.8 ÷ 2 = 4.9 m
Area of the circle = πr2 = 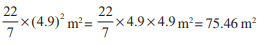
Example 19 The adjoining figure shows two circles with the same centre. The radius of the larger circle is
10 cm and the radius of the smaller circle is 4 cm.

Find: (a) the area of the larger circle
(b) the area of the smaller circle
(c) the shaded area between the two circles. (π = 3.14)
Solution
(a) Radius of the larger circle = 10 cm
So, area of the larger circle = πr2
= 3.14 × 10 × 10 = 314 cm2
(b) Radius of the smaller circle = 4 cm
Area of the smaller circle = πr2
= 3.14 × 4 × 4 = 50.24 cm2
(c) Area of the shaded region = (314 – 50.24) cm2 = 263.76 cm2
Exercise 11.3
1. Find the circumference of the circles with the following radius: (Take π = 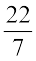 )
)
(a) 14 cm (b) 28 mm (c) 21 cm

2. Find the area of the following circles, given that:
(a) radius = 14 mm (Take π = 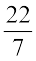 ) (b) diameter = 49 m
) (b) diameter = 49 m
(c) radius = 5 cm
3. If the circumference of a circular sheet is 154 m, find its radius. Also find the area of the sheet. (Take π = 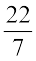 )
)
4. A gardener wants to fence a circular garden of diameter 21m. Find the length of the rope he needs to purchase, if he makes 2 rounds of fence. Also find the cost of the rope, if it costs ₹ 4 per meter. (Take π = 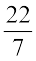 )
)
5. From a circular sheet of radius 4 cm, a circle of radius 3 cm is removed. Find the area of the remaining sheet. (Take π = 3.14)
6. Saima wants to put a lace on the edge of a circular table cover of diameter 1.5 m. Find the length of the lace required and also find its cost if one meter of the lace costs ₹ 15. (Take π = 3.14)
7. Find the perimeter of the adjoining figure, which is a semicircle including its diameter.
8. Find the cost of polishing a circular table-top of diameter 1.6 m, if the rate of polishing is ₹ 15/m2. (Take π = 3.14)
9. Shazli took a wire of length 44 cm and bent it into the shape of a circle. Find the radius of that circle. Also find its area. If the same wire is bent into the shape of a square, what will be the length of each of its sides? Which figure encloses more area, the circle or the square? (Take π = 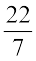 )
)
10. From a circular card sheet of radius 14 cm, two circles of radius 3.5 cm and a rectangle of length 3 cm and breadth 1cm are removed. (as shown in the adjoining figure). Find the area of the remaining sheet. (Take π = 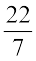 )
)
11. A circle of radius 2 cm is cut out from a square piece of an aluminium sheet of side
6 cm. What is the area of the left over aluminium sheet? (Take π = 3.14)
12. The circumference of a circle is 31.4 cm. Find the radius and the area of the circle?
(Take π = 3.14)
13. A circular flower bed is surrounded by a path 4 m wide. The diameter of the flower bed is 66 m. What is the area of this path? (π = 3.14) 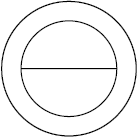
14. A circular flower garden has an area of 314 m2. A sprinkler at the centre of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take π = 3.14)
15. Find the circumference of the inner and the outer circles, shown in the adjoining figure? (Take π = 3.14)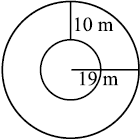
16. How many times a wheel of radius 28 cm must rotate to go 352 m? (Take π = 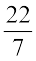 )
)
17. The minute hand of a circular clock is 15 cm long. How far does the tip of the minute hand move in 1 hour. (Take π = 3.14)
11.6 Conversion of Units
We know that 1 cm = 10 mm. Can you tell 1 cm2 is equal to how many mm2? Let us explore similar questions and find how to convert units while measuring areas to another unit.

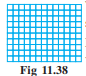
Draw a square of side 1cm (Fig 11.38), on a graph sheet.
You find that this square of side 1 cm will be divided into 100 squares, each of side 1 mm.
Area of a square of side 1cm = Area of 100 squares, of each side 1mm.
Therefore, 1 cm2 = 100 × 1 mm2
or 1 cm2 = 100 mm2
Similarly, 1 m2 = 1 m × 1 m
= 100 cm × 100 cm (As 1 m = 100 cm)
= 10000 cm2
Now can you convert 1 km2 into m2?
In the metric system, areas of land are also measured in hectares [written “ha” in short].
A square of side 100 m has an area of 1 hectare.
So, 1 hectare = 100 × 100 m2 = 10,000 m2
When we convert a unit of area to a smaller unit, the resulting number of units will be bigger.
For example, 1000 cm2 = 1000 × 100 mm2
= 100000 mm2
But when we convert a unit of area to a larger unit, the number of larger units will be smaller.
For example, 1000 cm2 = 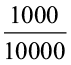 m2 = 0.1 m2
m2 = 0.1 m2
Try These
Convert the following:
(i) 50 cm2 in mm2 (ii) 2 ha in m2 (iii) 10 m2 in cm2 (iv) 1000 cm2 in m2
11.7 Applications
You must have observed that quite often, in gardens or parks, some space is left all around in the form of path or in between as cross paths. A framed picture has some space left all around it.
We need to find the areas of such pathways or borders when we want to find the cost of making them.
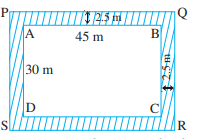
Example 20 A rectangular park is 45 m long and 30 m wide. A path 2.5 m wide is constructed outside the park. Find the area of the path.
Solution Let ABCD represent the rectangular park and the shaded region represent the path 2.5 m wide.
To find the area of the path, we need to find (Area of rectangle PQRS – Area of rectangle ABCD).
We have, PQ = (45 + 2.5 + 2.5) m = 50 m
PS = (30 + 2.5 + 2.5) m = 35 m
Area of the rectangle ABCD = l × b = 45 × 30 m2 = 1350 m2
Area of the rectangle PQRS = l × b = 50 × 35 m2 = 1750 m2
Area of the path = Area of the rectangle PQRS − Area of the rectangle ABCD
= (1750 − 1350) m2 = 400 m2
Example 21 A path 5 m wide runs along inside a square park of side
100 m. Find the area of the path. Also find the cost of
cementing it at the rate of ₹ 250 per 10 m2.
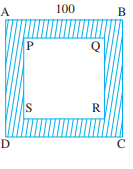
Solution Let ABCD be the square park of side 100 m. The shaded region represents the path 5 m wide.
PQ = 100 – (5 + 5) = 90 m
Area of square ABCD = (side)2 = (100)2 m2 = 10000 m2
Area of square PQRS = (side)2 = (90)2 m2 = 8100 m2
Therefore, area of the path = (10000 − 8100) m2 = 1900 m2
Cost of cementing 10 m2 = ₹ 250
Therefore, cost of cementing 1 m2 = ₹ 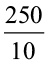
So, cost of cementing 1900 m2 = ₹
= ₹ 47,500
Example 22 Two cross roads, each of width 5 m, run at right angles through the centre of a rectangular park of length 70 m and breadth 45 m and parallel to its sides. Find the area of the roads. Also find the cost of constructing the roads at the rate of ₹ 105 per m2.
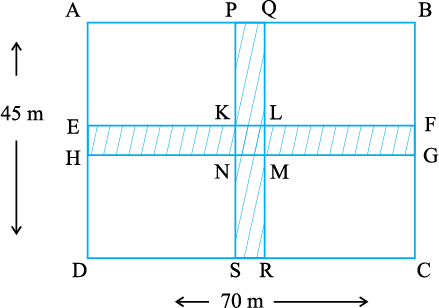
Solution Area of the cross roads is the area of shaded portion, i.e., the area of the rectangle PQRS and the area of the rectangle EFGH. But while doing this, the area of the square KLMN is taken twice, which is to be subtracted.
Now, PQ = 5 m and PS = 45 m
EH = 5 m and EF = 70 m
KL = 5 m and KN = 5 m
Area of the path = Area of the rectangle PQRS + area of the rectangle EFGH – Area of the square KLMN
= PS × PQ + EF × EH – KL × KN
= (45 × 5 + 70 × 5 − 5 × 5) m2
= (225 + 350 − 25) m2 = 550 m2
Cost of constructing the path = ₹ 105 × 550 = ₹ 57,750
Exercise 11.4
1. A garden is 90 m long and 75 m broad. A path 5 m wide is to be built outside and around it. Find the area of the path. Also find the area of the garden in hectare.
2. A 3 m wide path runs outside and around a rectangular park of length 125 m and breadth 65 m. Find the area of the path.
3. A picture is painted on a cardboard 8 cm long and 5 cm wide such that there is a margin of 1.5 cm along each of its sides. Find the total area of the margin.
4. A verandah of width 2.25 m is constructed all along outside a room which is 5.5 m long and 4 m wide. Find:
(i) the area of the verandah.
(ii) the cost of cementing the floor of the verandah at the rate of ₹ 200 per m2.
5. A path 1 m wide is built along the border and inside a square garden of side 30 m. Find:
(i) the area of the path
(ii) the cost of planting grass in the remaining portion of the garden at the rate of
₹ 40 per m2.
6. Two cross roads, each of width 10 m, cut at right angles through the centre of a rectangular park of length 700 m and breadth 300 m and parallel to its sides. Find the area of the roads. Also find the area of the park excluding cross roads. Give the answer in hectares.
7. Through a rectangular field of length 90 m and breadth 60 m, two roads are constructed which are parallel to the sides and cut each other at right angles through the centre of the fields. If the width of each road is 3 m, find
(i) the area covered by the roads.
(ii) the cost of constructing the roads at the rate of ₹ 110 per m2.
8. Pragya wrapped a cord around a circular pipe of radius 4 cm (adjoining figure) and cut off the length required of the cord. Then she wrapped it around a square box of side 4 cm (also shown). Did she have any cord left? (π = 3.14)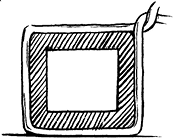
9. The adjoining figure represents a rectangular lawn with a circular flower bed in the middle. Find:

(i) the area of the whole land (ii) the area of the flower bed
(iii) the area of the lawn excluding the area of the flower bed
(iv) the circumference of the flower bed.
10. In the following figures, find the area of the shaded portions:
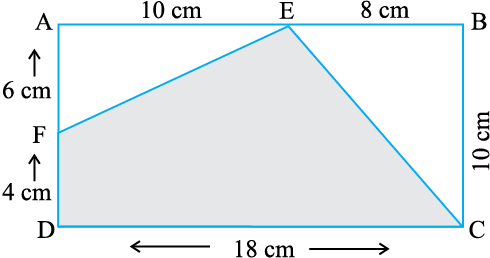
(i)

(ii)
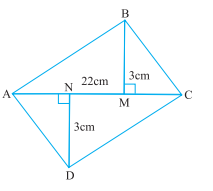
11. Find the area of the quadrilateral ABCD.
Here, AC = 22 cm, BM = 3 cm,
DN = 3 cm, and
BM ⊥ AC, DN ⊥ AC
What have we Discussed?
1. Perimeter is the distance around a closed figure whereas area is the part of plane occupied by the closed figure.
2. We have learnt how to find perimeter and area of a square and rectangle in the earlier class. They are:
(a) Perimeter of a square = 4 × side
(b) Perimeter of a rectangle = 2 × (length + breadth)
(c) Area of a square = side × side
(d) Area of a rectangle = length × breadth
3. Area of a parallelogram = base × height
4. Area of a triangle = 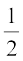 (area of the parallelogram generated from it)
(area of the parallelogram generated from it)
= 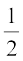 × base × height
× base × height
5. The distance around a circular region is known as its circumference.
Circumference of a circle = πd, where d is the diameter of a circle and 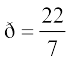
or 3.14 (approximately).
6. Area of a circle = πr2, where r is the radius of the circle.
7. Based on the conversion of units for lengths, studied earlier, the units of areas can also be converted:
1 cm2 = 100 mm2, 1 m2 = 10000 cm2 , 1 hectare = 10000 m2.