Table of Contents
Chapter 11
Constructions
11.1 Introduction
In earlier chapters, the diagrams, which were necessary to prove a theorem or solving exercises were not necessarily precise. They were drawn only to give you a feeling for the situation and as an aid for proper reasoning. However, sometimes one needs an accurate figure, for example - to draw a map of a building to be constructed, to design tools, and various parts of a machine, to draw road maps etc. To draw such figures some basic geometrical instruments are needed. You must be having a geometry box which contains the following:
(i) A graduated scale, on one side of which centimetres and millimetres are marked off and on the other side inches and their parts are marked off.
(ii) A pair of set - squares, one with angles 90°, 60° and 30° and other with angles 90°, 45° and 45°.
(iii) A pair of dividers (or a divider) with adjustments.
(iv) A pair of compasses (or a compass) with provision of fitting a pencil at one end.
(v) A protractor.
Normally, all these instruments are needed in drawing a geometrical figure, such as a triangle, a circle, a quadrilateral, a polygon, etc. with given measurements. But a geometrical construction is the process of drawing a geometrical figure using only two instruments – an ungraduated ruler, also called a straight edge and a compass. In construction where measurements are also required, you may use a graduated scale and protractor also. In this chapter, some basic constructions will be considered. These will then be used to construct certain kinds of triangles.
11.2 Basic Constructions
In Class VI, you have learnt how to construct a circle, the perpendicular bisector of a line segment, angles of 30°, 45°, 60°, 90° and 120°, and the bisector of a given angle, without giving any justification for these constructions. In this section, you will construct some of these, with reasoning behind, why these constructions are valid.
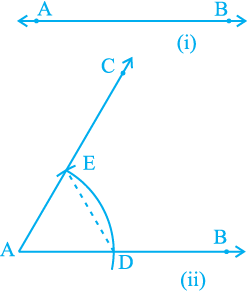
Construction 11.1 : To construct the bisector of a given angle.
Given an angle ABC, we want to construct its bisector.
Steps of Construction :
1. Taking B as centre and any radius, draw an arc to intersect the rays BA and BC, say at E and D respectively [see Fig.11.1(i)].
2. Next, taking D and E as centres and with the radius more than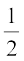 
DE, draw arcs to intersect each other, say at F.
3. Draw the ray BF [see Fig.11.1(ii)].This ray BF is the required bisector of the angle ABC.
Fig. 11.1
Let us see how this method gives us the required angle bisector.
Join DF and EF.
In triangles BEF and BDF,
BE = BD (Radii of the same arc)
EF = DF (Arcs of equal radii)
BF = BF (Common)
Therefore, ∆BEF ≅ ∆BDF (SSS rule)
This gives ∠ EBF = ∠ DBF (CPCT)
Construction 11.2 : To construct the perpendicular bisector of a given line segment.
Given a line segment AB, we want to construct its perpendicular bisector.
Steps of Construction :
1. Taking A and B as centres and radius more than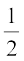 
AB, draw arcs on both sides of the line segment AB (to intersect each other).
2. Let these arcs intersect each other at P and Q. Join PQ (see Fig.11.2).
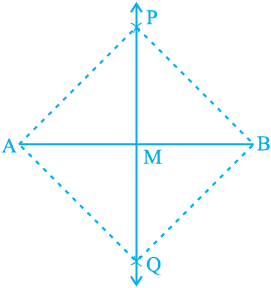
Fig. 11.2
3. Let PQ intersect AB at the point M. Then line PMQ is the required perpendicular bisector of AB.
Let us see how this method gives us the perpendicular bisector of AB.
Join A and B to both P and Q to form AP, AQ, BP and BQ.
In triangles PAQ and PBQ,
AP = BP (Arcs of equal radii)
AQ = BQ (Arcs of equal radii)
PQ = PQ (Common)
Therefore, ∆ PAQ ≅ ∆ PBQ (SSS rule)
So, ∠ APM = ∠ BPM (CPCT)
Now in triangles PMA and PMB,
AP = BP (As before)
PM = PM (Common)
∠ APM = ∠ BPM (Proved above)
Therefore, ∆ PMA ≅ ∆ PMB (SAS rule)
So, AM = BM and ∠ PMA = ∠ PMB (CPCT)
As ∠ PMA + ∠ PMB = 180° (Linear pair axiom),
we get
∠ PMA = ∠ PMB = 90°.
Therefore, PM, that is, PMQ is the perpendicular bisector of AB.
Construction 11.3 : To construct an angle of 600 at the initial point of a given ray.
Let us take a ray AB with initial point A [see Fig. 11.3(i)]. We want to construct a ray AC such that ∠ CAB = 60°. One way of doing so is given below.
Steps of Construction :
1. Taking A as centre and some radius, draw an arc of a circle, which intersects AB, say at a point D.
2. Taking D as centre and with the same radius as before, draw an arc intersecting the previously drawn arc, say at a point E.
3. Draw the ray AC passing through E[see Fig 11.3 (ii)].
Then ∠ CAB is the required angle of 60°. Now, let us see how this method gives us the required angle of 60°.
Join DE.
Then, AE = AD = DE (By construction)
Therefore, ∆ EAD is an equilateral triangle and the ∠ EAD, which is the same as ∠ CAB is equal to 60°.
EXERCISE 11.1
1. Construct an angle of 900 at the initial point of a given ray and justify the construction.
2. Construct an angle of 450 at the initial point of a given ray and justify the construction.
3. Construct the angles of the following measurements:
(i) 30° (ii) 22 
° (iii) 15°
4. Construct the following angles and verify by measuring them by a protractor:
(i) 75° (ii) 105° (iii) 135°
5. Construct an equilateral triangle, given its side and justify the construction.
11.3 Some Constructions of Triangles
So far, some basic constructions have been considered. Next, some constructions of triangles will be done by using the constructions given in earlier classes and given above. Recall from the Chapter 7 that SAS, SSS, ASA and RHS rules give the congruency of two triangles. Therefore, a triangle is unique if : (i) two sides and the included angle is given, (ii) three sides are given, (iii) two angles and the included side is given and, (iv) in a right triangle, hypotenuse and one side is given. You have already learnt how to construct such triangles in Class VII. Now, let us consider some more constructions of triangles. You may have noted that at least three parts of a triangle have to be given for constructing it but not all combinations of three parts are sufficient for the purpose. For example, if two sides and an angle (not the included angle) are given, then it is not always possible to construct such a triangle uniquely.
Construction 11.4 : To construct a triangle, given its base, a base angle and sum of other two sides.
Given the base BC, a base angle, say ∠ B and the sum AB + AC of the other two sides of a triangle ABC, you are required to construct it.
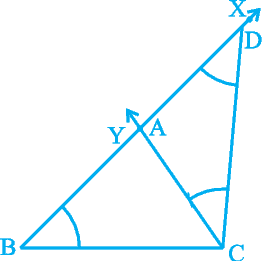
Fig. 11.4
Steps of Construction :
1. Draw the base BC and at the point B make an angle, say XBC equal to the given angle.
2. Cut a line segment BD equal to AB + AC from the ray BX.
3. Join DC and make an angle DCY equal to ∠ BDC.
4. Let CY intersect BX at A (see Fig. 11.4).
Then, ABC is the required triangle.
Let us see how you get the required triangle.
Base BC and ∠ B are drawn as given. Next in triangle ACD,
∠ ACD = ∠ ADC (By construction)
Therefore, AC = AD and then
AB = BD – AD = BD – AC
AB + AC = BD
Alternative method :
Follow the first two steps as above. Then draw perpendicular bisector PQ of CD to intersect BD at a point A (see Fig 11.5).Join AC. Then ABC is the required triangle. Note that A lies on the perpendicular bisector of CD, therefore AD = AC.
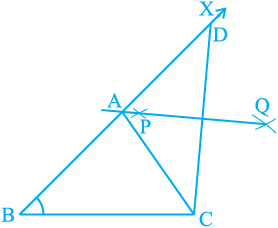
Fig. 11.5
Remark : The construction of the triangle is not possible if the sum AB + AC ≤ BC.
Construction 11.5 : To construct a triangle given its base, a base angle and the difference of the other two sides.
Given the base BC, a base angle, say ∠ B and the difference of other two sides
AB – AC or AC – AB, you have to construct the triangle ABC. Clearly there are following two cases:
Case (i) : Let AB > AC that is AB – AC is given.
Steps of Construction :
1. Draw the base BC and at point B make an angle say XBC equal to the given angle.
2. Cut the line segment BD equal to AB – AC from ray BX.
3. Join DC and draw the perpendicular bisector, say PQ of DC.
4. Let it intersect BX at a point A. Join AC
(see Fig. 11.6).
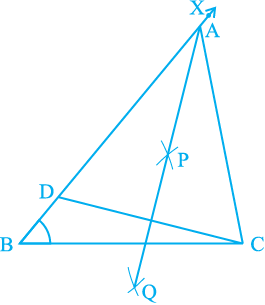
Fig. 11.6
Then ABC is the required triangle.
Let us now see how you have obtained the required triangle ABC.
Base BC and ∠ B are drawn as given. The point A lies on the perpendicular bisector of DC. Therefore,
AD = AC
So, BD = AB – AD = AB – AC.
Case (ii) : Let AB < AC that is AC – AB is given.
Steps of Construction :
1. Same as in case (i).
2. Cut line segment BD equal to AC – AB from the line BX extended on opposite side of line segment BC.
3. Join DC and draw the perpendicular bisector, say PQ of DC.
4. Let PQ intersect BX at A. Join AC (see Fig. 11.7).
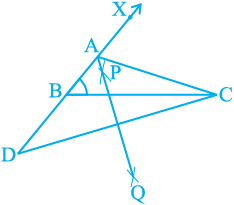
Fig. 11.7
Then, ABC is the required triangle.
You can justify the construction as in case (i).
Construction 11.6 : To construct a triangle, given its perimeter and its two base angles.
Given the base angles, say ∠ B and ∠ C and BC + CA + AB, you have to construct the triangle ABC.
Steps of Construction :
1. Draw a line segment, say XY equal to BC + CA + AB.
2. Make angles LXY equal to ∠ B and MYX equal to ∠ C.
3. Bisect ∠ LXY and ∠ MYX. Let these bisectors intersect at a point A
[see Fig. 11.8(i)].
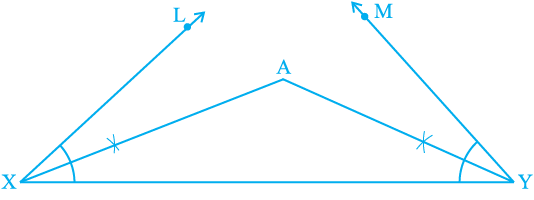
Fig. 11.8 (i)
4. Draw perpendicular bisectors PQ of AX and RS of AY.
5. Let PQ intersect XY at B and RS intersect XY at C. Join AB and AC
[see Fig 11.8(ii)].
Fig. 11.8 (ii)
Then ABC is the required triangle. For the justification of the construction, you observe that, B lies on the perpendicular bisector PQ of AX.
Therefore, XB = AB and similarly, CY = AC.
This gives BC + CA + AB = BC + XB + CY = XY.
Again ∠ BAX = ∠ AXB (As in ∆ AXB, AB = XB) and
∠ ABC = ∠ BAX + ∠ AXB = 2 ∠ AXB = ∠ LXY
Similarly, ∠ ACB = ∠ MYX as required.
Example 1 : Construct a triangle ABC, in which ∠ B = 60°, ∠ C = 45° and AB + BC + CA = 11 cm.
Steps of Construction :
1. Draw a line segment PQ = 11 cm.( = AB + BC + CA).
2. At P construct an angle of 60° and at Q, an angle of 45°.
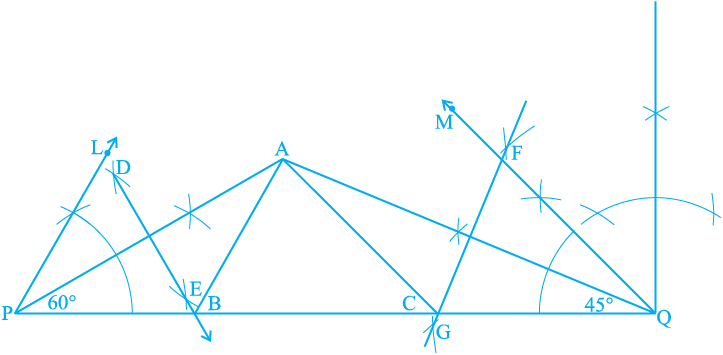
Fig. 11.9
3. Bisect these angles. Let the bisectors of these angles intersect at a point A.
4. Draw perpendicular bisectors DE of AP to intersect PQ at B and FG of AQ to intersect PQ at C.
5. Join AB and AC (see Fig. 11.9).
Then, ABC is the required triangle.
Exercise 11.2
1. Construct a triangle ABC in which BC = 7cm, ∠ B = 75° and AB + AC = 13 cm.
2. Construct a triangle ABC in which BC = 8cm, ∠ B = 45° and AB – AC = 3.5 cm.
3. Construct a triangle PQR in which QR = 6cm, ∠ Q = 60° and PR – PQ = 2cm.
4. Construct a triangle XYZ in which ∠ Y = 30°, ∠ Z = 90° and XY + YZ + ZX = 11 cm.
5. Construct a right triangle whose base is 12cm and sum of its hypotenuse and other side is 18 cm.
11.4 Summary
In this chapter, you have done the following constructions using a ruler and a compass:
1. To bisect a given angle.
2. To draw the perpendicular bisector of a given line segment.
3. To construct an angle of 60° etc.
4. To construct a triangle given its base, a base angle and the sum of the other two sides.
5. To construct a triangle given its base, a base angle and the difference of the other two sides.
6. To construct a triangle given its perimeter and its two base angles.