Table of Contents
Introduction To Aerial Photographs
We are familiar with photographs taken with normal cameras. These photographs provide us with a view of the object similar to the way we see them with our own eyes. In other words, we get a horizontal perspective of the objects photographed. For example, a photograph of a part of settlement will provide us a perspective the way it appears to us when we look at it (Fig. 6.1).

Figure 6.1 Terrestrial photograph of Mussorrie town
Suppose we want to take a ‘bird’s eye view’ of similar features, then we have to place ourselves somewhere in the air. When we do so and look down, we get a very different perspective. This perspective, which we get in aerial photographs, is termed as aerial perspective (Fig. 6.2).

Figure 6.2 Bird’s Eye View of Tehri Town, Uttarakhand
The photographs taken from an aircraft or helicopter using a precision camera are termed aerial photographs. The photographs so obtained have been found to be indispensable tools in the topographical mapping and interpretation of the images of the objects.
Glossary
Aerial Camera : A precision camera specifically designed for use in aircrafts.
Aerial Film : A roll film with high sensitivity, high intrinsic resolution power and dimensionally stable emulsion support.
Aerial Photography : Art, science and technology of taking aerial photographs from an air-borne platform.
Aerial Photograph : A photograph taken from an air-borne platform using a precision camera.
Fiducial Marks : Index marks, rigidly connected at the central or corner edges of the camera body. When the film is exposed, these marks appear on the film negative .
Forward Overlap : The common area on two successive photographs in the flight direction. It is usually expressed in per cent.
Image Interpretation : An act of identifying the images of the objects and judging their relative significance.
Nadir Point : The foot of the perpendicular drawn from the camera lens centre on the ground plane.
Principal Point : The foot of the perpendicular drawn from the camera lens centre on the photo plane.
Principal Distance : The perpendicular distance from the perspective centre to the plane of the photograph.
Perspective Centre : The point of origin (perspective centre) of the bundle of light rays.
Photogrammetry : The science and technology of taking reliable measurements from aerial photographs.
Uses of Aerial Photographs
Aerial photographs are used in topographical mapping and interpretation. These two different uses have led to the development of photogrammetry and photo/image interpretation as two independent but related sciences.
Photogrammetry: It refers to the science and technology of making reliable measurements from aerial photographs. The principles used in photogrammetry facilitate precise measurements related to the length, breadth and height from such photographs. Hence, they are used as the data source for creating and updating topographic maps.
The development of aerial photography in India is briefly given in Box 6.I.
Box 6.1 Aerial Photography in India
Aerial photography in India goes back to 1920 when large-scale aerial photographs of Agra city were obtained. Subsequently, Air Survey Party of the Survey of India took up aerial survey of Irrawaddy Delta forests,
which was completed during 1923–24. Subsequently, several similar surveys were carried out and advanced methods of mapping from aerial photographs were used. Today, aerial photography in India is carried out for the entire country under the overall supervision of the Directorate of Air Survey (Survey of India) New Delhi. Three flying agencies, i.e. Indian Air Force, Air Survey Company, Kolkata and National Remote Sensing Agency, Hyderabad have been officially authorised to take aerial photographs in India.
The procedure for indenting aerial photographs for educational purposes could be made with APFPS Party No. 73, Directorate of Air Survey, Survey of India, West Block IV, R. K. Puram, New Delhi.
Image Interpretation: It is an art of identifying images of objects and judging their relative significance. The principles of image interpretation are applied to obtain qualitative information from the aerial photographs such as land use/land cover, topographical forms, soil types, etc. A trained interpreter can thus utilise aerial photographs to analyse the land-use changes.
Advantages of Aerial Photography
The basic advantages that aerial photographs offer over ground based observation are :
a. Improved vantage point: Aerial photography provides a bird’s eye view of large areas, enabling us to see features of the earth surface in their spatial context.
b. Time freezing ability: An aerial photograph is a record of the surface features at an instance of exposure. It can, therefore, be used as a historical record.
c. Broadened Sensitivity: The sensitivity of the film used in taking aerial photographs is relatively more than the sensitivity of the human eyes. Our eyes perceive only in the visible region of the electromagnetic spectrum, i.e. 0.4 to 0.7 µm whereas the sensitivity of the film ranges from 0.3 to 0.9 µm.
d. Three dimensional perspective: Aerial photographs are normally taken with uniform exposure interval that enables us in obtaining stereo pair of photographs. Such a pair of photographs helps us in getting a three-dimensional view of the surface photographed.
Types of Aerial Photographs
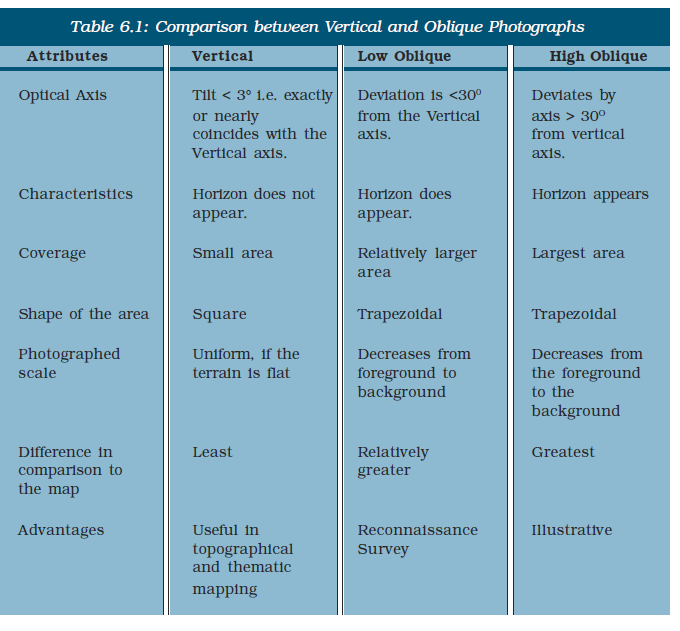
The aerial photographs are classified on the basis of the position of the camera axis, scale, angular extent of coverage and the film used. The types of the aerial photographs based on the position of optical axis and the scale are given below :d.
a. Types of Aerial Photographs Based on the Position of the Cameral Axis: On the basis of the position of the camera axis, aerial photographs are classified into the following types :
(i) Vertical photographs
(ii) Low oblique photographs
(iii) High oblique photographs
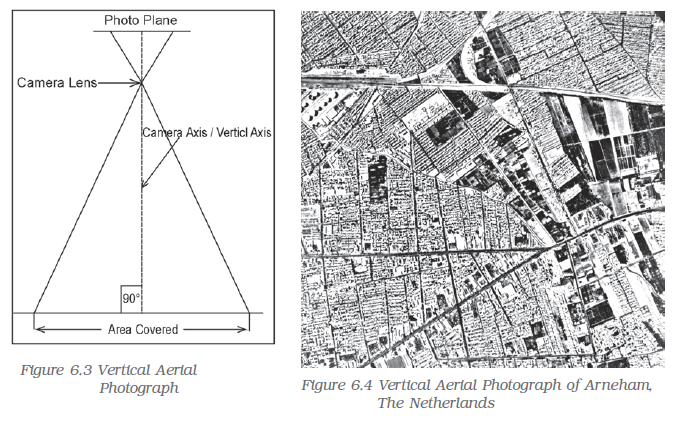
(i) Vertical Photographs: While taking aerial photographs, two distinct axes are formed from the camera lens centre, one towards the ground plane and the other towards the photo plane. The perpendicular dropped from the camera lens centre to the ground plane is termed as the vertical axis, whereas the plumb line drawn from the lens centre to the photo plane is known as the photographic/optical axis. When the photo plane is kept parallel to the ground plane, the two axes also coincide with each other. The photograph so obtained is known as vertical aerial photograph (Figures 6.3 and 6.4).
However, it is normally very difficult to achieve perfect parallelism between the two planes due to the fact that the aircraft flies over the curved surface of the earth. The photographic axis, therefore, deviates from the vertical axis. If such a deviation is within the range of plus or minus 3o, the near-vertical aerial photographs are obtained. Any photography with an unintentional deviation of more than 3o in the optical axis from the vertical axis is known as a tilted photograph.
(ii) Low Oblique: An aerial photograph taken with an intentional deviation of 15° to 30° in the camera axis from the vertical axis is referred to as the low oblique photograph (Figures 6.5 and 6.6). This kind of photograph is often used in reconnaissance surveys.

(iii) High Oblique: The high oblique are photographs obtained when the camera axis is intentionally inclined about 60° from the vertical axis (Figure 6.7). Such photography is useful in reconnaissance surveys.
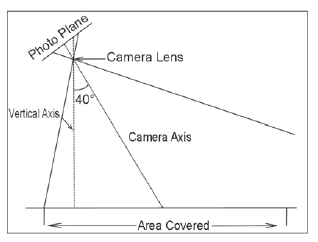
Figure 6.7 High Oblique Photograph
Table 6.1 provides a comparison between vertical and oblique photographs.
b) Types of Aerial Photographs Based on Scale: The aerial photographs may also be classified on the basis of the scale of photograph into three types.
(i) Large Scale Photographs: When the scale of an aerial photograph is 1 : 15,000 and larger, the photography is classified as large-scale photograph (Fig. 6.8).

Figure 6.8 1 : 5000 Photograph of Arnehem
(ii) Medium Scale Photographs: The aerial photographs with a scale ranging between 1 : 15,000 and 1 : 30,000 are usually treated as medium scale photographs (Fig. 6.9).

Figure 6.9 1 : 20,000 Photograph of Arnehem
(iii) Small Scale Photographs: The photographs with the scale being smaller than 1 : 30,000, are referred to as small scale photographs (Fig. 6.10).

Figure 6.10 1 : 40,000 Photograph of Arnehem
Geometry of an Aerial Photograph
To understand the geometry of an aerial photograph, it is important to appreciate the orientation of the photograph with respect to the ground, i.e. the way the rays connect or ‘project’ onto the ground in relation to the ground representation (photograph or map). The following three examples of such projection would be useful in understanding the problem.
Parallel Projection: In this projection, the projecting rays are parallel but not necessarily perpendicular. The triangle ABC is projected on LL1 as triangle abc (figure 6.11).
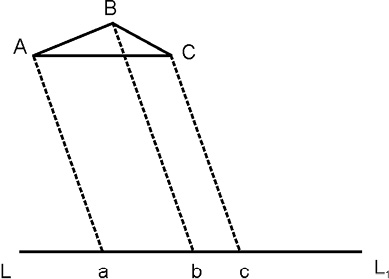
Figure 6.11 Parallel Projection
Orthogonal Projection: This is a special case of parallel projections. Maps are orthogonal projections of the ground. The advantage of this projection is that the distances, angles or areas on the plane are independent of the elevation differences of the objects. Figure 6.12 is an example of orthogonal projection where the projecting rays are perpendicular to the line LL1.
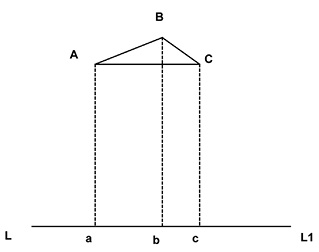
Figure 6.12 Orthogonal projection
Central Projection: Figure 6.13 shows an example of Central Projection. The projecting rays Aa, Bb and Cc pass through a common point O, which is called the perspective Centre. The image projected by a lens is treated like a central projection.
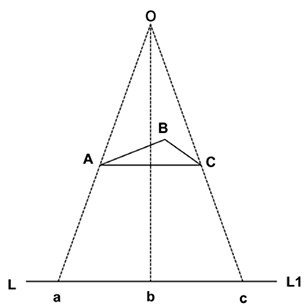
Figure 6.13 Central Projection
An aerial photograph, as discussed earlier is a central projection. In an absolutely vertical flat terrain the aerial photograph will be geometrically the same as the corresponding map of the area. However, because of the tilt of the photograph and relief variations of the ground photographed, an aerial photograph differs geometrically from the map of the corresponding area.
As shown in Figure 6.14, S is the camera lens centre. The bundle of light rays coming from the ground plane converge at this point and diverge from there towards the negative (photo) plane to form images of the objects. Thus, the central projection is characterised by the fact that all straight lines joining corresponding points, i.e. straight lines joining object points to their corresponding image points pass through one point. Figure 6.14 illustrates this relationship. Straight lines AAi, BBi, CCi and DDi join corresponding points on the ground photographed and the negative plane. For example, A on the ground and Ai on the negative plane (or ‘a’ on the positive plane) is a line joining corresponding points which pass through the camera lens centre. If we draw a perpendicular from S following the camera axis onto the negative plane, the point where this perpendicular meets the negative is known as the principal point (P in Fig. 6.14). If we extend the same line to the ground, it would meet the target (photographed ground) plane at PG, i.e. the ground principal point. Similarly, if we draw a vertical line (plumb line as indicated by the direction of gravity) through S, it will meet the photo negative at a point known as the nadir point and on the ground as the ground nadir point. Observe from figures 6.3, 6.5 and 6.7 that the plumb line and the camera axis are coincident for a vertical photograph while they are separable in case of an oblique or a tilted photograph. Thus in case of a vertical photograph, the principal and the nadir points also coincide with one another. For an oblique photograph, the angle between the camera axis and the plumb line is the tilt angle. Figure 6.14 shows both the positive and the negative planes of a vertical photograph. The geometry of the positive and the negative planes are identical.
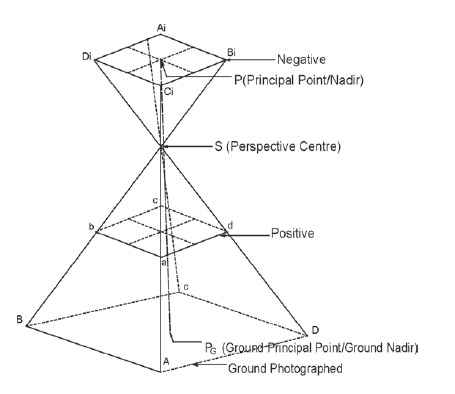
Figure 6.14 Geometry of Vertical Photograph
It needs to be understood here that SP, i.e. the perpendicular distance between the camera lens and the negative plane is known as the focal length. On the other hand, SPG, i.e., the perpendicular distance between the camera lens and the ground photographed is known as the flying height
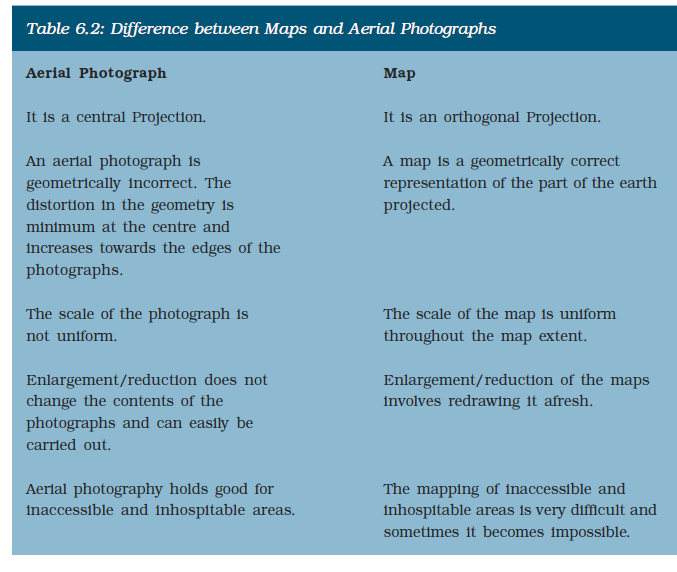
Difference between a Map and an Aerial Photograph
A map cannot be directly traced out of an aerial photograph. The reason is that there is a basic difference in the planimetry (projection) and perspective of a map and an aerial photograph. The difference is given in Table 6.2.
Even vertical aerial photographs do not have a consistent scale unless they have been taken of a flat terrain. Aerial photographs need to be transformed from perspective view to the planimetric view before they can be used as map substitute. Such transformed photographs are known as orthophotos.
Scale of Aerial Photograph
You are already familiar with the concept of a map scale (See Chapter 2). The concept of scale for aerial photographs is much the same as that of a map. Scale is the ratio of a distance on an aerial photograph the distance between the same two places on the ground in the real world. It can be expressed in unit equivalents like 1 cm= 1,000 km(or 12,000 inches) or as a representative fraction (1:100,000).
Scale determines what objects would be visible, the accuracy of estimates and how certain features will appear. When conducting an analysis that is based on air photos, it will sometimes be necessary to make estimates regarding the number of objects, the area covered by a certain amount of material or it may be possible to identify certain features based on their length. To determine this dimension during air photo interpretation, it will be necessary to make estimates of lengths and areas, which require knowledge of the photo scale. There are three methods to compute the scale of an aerial photograph using different sets of information.
Method 1: By Establishing Relationship between Photo Distance and Ground Distance : If additional information like ground distances of two identifiable points in an aerial photograph is available, it is fairly simple to work out the scale of a vertical photograph. Provided that the corresponding ground distances (Dg) are known for which the distances on an aerial photograph (Dp) are measured. in such cases, the scale of an aerial photograph will be measured as a ratio of the two, i.e. Dp/ Dg.
Problem 6.1 The distance between two points on an aerial photograph is measured as 2 centimetres. The known distance between the same two points on the ground is 1 km. Compute the scale of the aerial photograph (Sp).
Solution
Sp = Dp : Dg
= 2 cm : 1 km
= 2cm : 1 x 100,000 cm
= 1 : 100,000/2 = 50,000 cm
= 1 unit represents 50,000 units
Therefore, Sp = 1 : 50,000
Method 2: By Establishing Relationship between Photo Distance and Map Distance: As we know, the distances between different points on the ground are not always known. However, if a reliable map is available for the area shown on an aerial photograph, it can be used to determine the photo scale. In other words, the distances between two points identifiable both on a map and the aerial photograph enable us to compute the scale of the aerial photograph (Sp). The relationship between the two distances may be expressed as under :
(Photo scale : Map scale) = (Photo distance : Map distance)
We can derive
Photo scale (Sp) = Photo distance (Dp) : Map distance (Dm) x Map scale factor (msf)
Problem 6.2 The distance measured between two points on a map is 2 cm. The corresponding distance on an aerial photograph is 10 cm. Calculate the scale of the photograph when the scale of the map is 1: 50,000.
Solution
Sp = Dp : Dm x msf
Or = 10 cm : 2 cm x 50,000
Or = 10 cm : 100,000 cm
Or = 1 : 100,000/10 = 10,000 cm
Or = 1 unit represents 10,000 units
Therefore, Sp = 1 : 10,000
Method 3: By Establishing Relationship between Focal Length (f) and Flying Height (H) of the Aircraft : If no additional information is available about the relative distances on photograph and ground/map, we can determine the photo-scale provided the information about the focal length of the camera (f) and the flying height of the aircraft (H) are known (Fig. 6.15). The photo scale so determined could be more reliable if the given aerial photograph is truly vertical or near vertical and the terrain photographed is flat. The focal length of the camera (f) and the flying height of the aircraft (H) are provided as marginal information on most of the vertical photographs (Box 6.2).
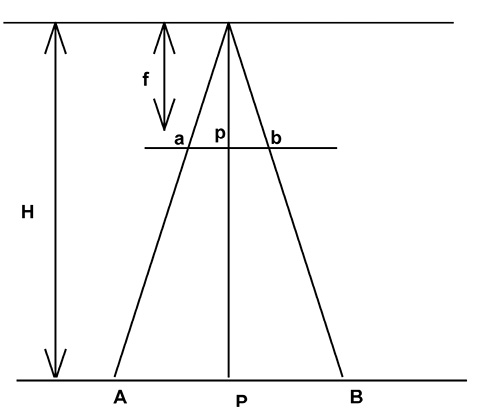
Figure 6.15 Focal Length of the Camera (f) and Flying Height of the Aircraft (H)
The Fig. 6.15 may be used to derive the photo-scale formula in the following way :
Focal Length (f) : Flying Height(H) =
Photo distance (Dp) : Ground distance (Dg)
Problem 6.3 Compute the scale of an aerial photograph when the flying height of the aircraft is 7500m and the focal length of the camera is 15cm.
Sp = f : H
Or Sp = 15 cm : 7,500 x 100 cm
Or Sp = 1 : 750,000/15
Therefore, Sp = 1 : 50,000
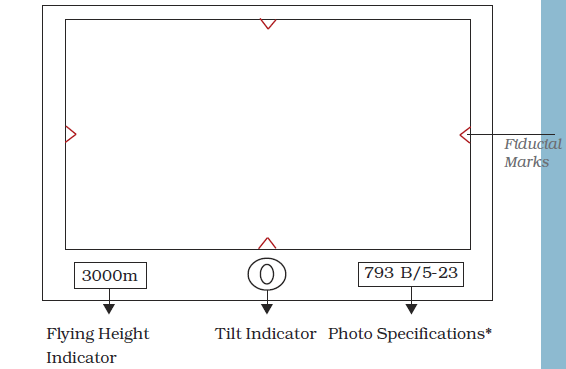
* 793 is a Photo Specification number maintained by the 73 APFPS Party of the Survey of India. B is the Flying Agency that carried out the present photography (In India three flying agencies are officially permitted to carry out aerial photography. They are the Indian Air Force, the Air Survey Company, Kolkata and the National Remote Sensing Agency, Hydrabad, identified on the aerial photographs as A, B and C respectively), 5 is the strip number and 23 is the photo number in strip 5.
Exercise
Multiple Choice Questions
1. In which of the following aerial photographs the horizon appears?
a. Vertical
b. Near-vertical
c. Low-oblique
d. High-oblique
2. In which of the following aerial photographs the Nadir and the principle points coincide?
a. Vertical
b. Near-vertical
c. Low-oblique
d. High-oblique
3. Which type of the following projections is used in aerial photographs?
a. Parallel
b. Orthogonal
c. Central
d. None of the above.
Short Questions
1. State any three advantages that an aerial photograph offers over ground based observations.
2. How is an aerial photograph taken?
3. Present a concise account of aerial photography in India.
4. Answer the following questions in about 125 words :
i) What are the two major uses of an aerial photograph? Elaborate.
ii) What are the different methods of scale determination?