Given the curve ay + x² = 7 and x³ = y, cut orthogonally at (1, 1),

ay + x² = 7

Differentiating on both sides with respect to x, we get

Applying the sum rule of differentiation and also the derivative of the constant is 0, so we get

Applying the power rule we get

The value of above derivative at (1,1), becomes

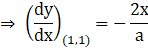
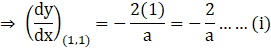
x³ = y

Differentiating on both sides with respect to x, we get

Applying the power rule we get

The value of above derivative at (1,1), becomes

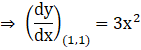
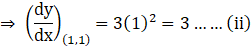
Now as these two curves cut orthogonally at (1,1), so

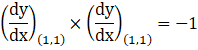
so from equation (i) and (ii), we get

⇒ a=6

Hence f the curve ay + x² = 7 and x³ = y, cut orthogonally at (1, 1), then the value of a is 6.

So the correct option is option D.