Given: (1 + i)²ⁿ = (1 – i)²ⁿ

Consider the given equation,

(1 + i)²ⁿ = (1 – i)²ⁿ

Now, rationalizing by multiply and divide by the conjugate of (1 – i)

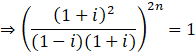
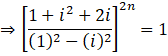
[(a + b)² = a² + b² + 2ab & (a – b)(a + b) = (a² – b²)]

[i² = -1]

⇒ (i)²ⁿ = 1

Now, i²ⁿ = 1 is possible if n = 2 because (i)²⁽²⁾ = i⁴ = (-1)⁴ = 1

So, the smallest positive integer n = 2