Find the modulus of each of the following complex numbers and hence express each of them in polar form: 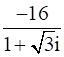
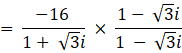
![]()
![]()
= 4![]() i - 4
i - 4
Let Z = 4![]() i - 4 = r(cosθ + isinθ)
i - 4 = r(cosθ + isinθ)
Now , separating real and complex part , we get
-4 = rcosθ ……….eq.1
![]() = rsinθ …………eq.2
= rsinθ …………eq.2
Squaring and adding eq.1 and eq.2, we get
64 = r2
Since r is always a positive no., therefore,
r = 8,
hence its modulus is 8.
now, dividing eq.2 by eq.1 , we get,
![]()
tanθ = -√3
Since ![]() ,
, ![]() and tanθ = -√3. therefore the θ lies in second quadrant.
and tanθ = -√3. therefore the θ lies in second quadrant.
Tanθ = -√3, therefore ![]()
Representing the complex no. in its polar form will be
![]() }
}
1