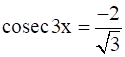
To Find: General solution.

(i) Given: sec 3x = -2

We know that sec cos = 1

So cos 3x =

Formula used: cos = cos = 2n , n I

By using above formula, we have

cos 3x = = -cos= cos= cos 3x = 2n x = , n I

So the general solution is x = , ,where n I

(ii) Given: cot 4x = -1

We know that tan cot = 1

So tan 4x = -1

Formula used: tan = tan = n , n I

By using above formula, we have

tan 4x = -1= tan 4x = n x = , n I

So general solution is x = (4n+3) ,where n I

(iii) Given: cosec 3x =

We know that cosec sin = 1

So sin 3x =

Formula used: sin = sin = n + (-1)ⁿ. , n I

By using above formula, we have

sin 3x = 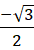= sin 3x= n +(-1)ⁿ . x= +(-1)ⁿ . , n I

So general solution is x = +(-1)ⁿ . , where n I