Given: Foci are (0, ±13), the conjugate axis is of the length 24

Need to find: The equation of the hyperbola.

Let, the equation of the hyperbola be:

The conjugate axis is of the length 24, i.e., 2b = 24

Therefore, b = 12

The foci are given at (0, ±13)

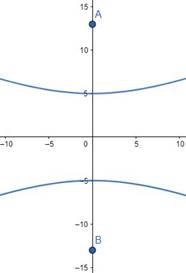
A and B are the foci.

That means, ae = 13, where e is the eccentricity.

We know that,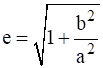

Therefore,

⇒

⇒

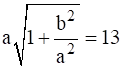
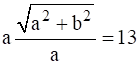
⇒ [Squaring both sides]

⇒ [As b = 12]

So, the equation of the hyperbola is,

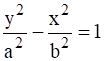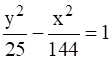⇒ [Answer]