Choose the correct answer If 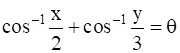, then 9x 2 – 12xy cos θ + 4y 2 is equal to

Question

Philoid · Accepted Answer

We are given with,

We need to find the value of 9x² – 12xy cos θ + 4y².

Using property of inverse trigonometry,

Take Left Hand Side (LHS) of:

Replace A by and B by .

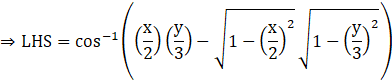
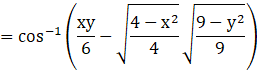
Further solving,

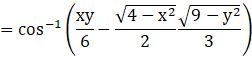
We shall equate LHS to RHS,

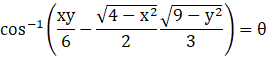
Taking cosine on both sides,

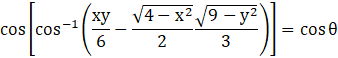
Using property of inverse trigonometry,

cos(cos^-1 A) = A

So,

By cross-multiplying,

⇒ xy - √(4 – x²) √(9 – y²) = 6 cos θ

Rearranging it,

⇒ xy – 6 cos θ = √(4 – x²) √(9 – y²)

Squaring on both sides,

⇒ [xy – 6 cos θ]² = [√(4 – x²) √(9 – y²)]²

Using algebraic identity,

(a – b)² = a² + b² – 2ab

⇒ (xy)² + (6 cos θ)² – 2(xy)(6 cos θ) = (4 – x²)(9 – y²)

⇒ x²y² + 36 cos² θ – 12xy cos θ = 36 – 9x² – 4y² + x²y²

⇒ x²y² – x²y² + 9x² – 12xy cos θ + 4y² = 36 – 36 cos² θ

⇒ 9x² – 12xy cos θ + 4y² = 36 (1 – cos² θ)

Using trigonometric identity,

sin² θ + cos² θ = 1

⇒ sin² θ = 1 – cos² θ

Substituting the value of (1 – cos² θ), we get

⇒ 9x² – 12xy cos θ + 4y² = 36 sin² θ

Book: RD Sharma - Mathematics (Volume 1)