By graphical method, the solution of linear programming problem
Maximize Z = 3x1 + 5x2
Subject to 3x1 + 2x2 ≤ 18
x1 ≤ 4
x2 ≤ 6
x1 ≥ 0, x2 ≥ 0, is
Given objective function is Z = 3x1 + 5x2
Subject to constraints:
3x1 + 2x2 ≤ 18
x1 ≤ 4
x2 ≤ 6
x1 ≥ 0, x2 ≥ 0
To solve this,
Consider the constraints as equations for a while, then we will have
3x1 + 2x2 = 18
x1 = 4
x2 = 6
x1 = 0, x2 = 0
Consider the 3x1 + 2x2 = 18, for graphing this, we can find the intercepts of this equation and then plot the line.
By dividing the whole equation with 18 we get,
![]()
![]()
From this equation we know that, x1 will intercept the line x1 = 0 at (6,0) and x2 will intercept the line x2 = 0 at (0,9)
Using these points to plot 3x1 + 2x2 = 18 in the graph, continue plotting the other equations, x1 = 4 , x2 = 6 , x1 = 0, x2 = 0 on he graph.
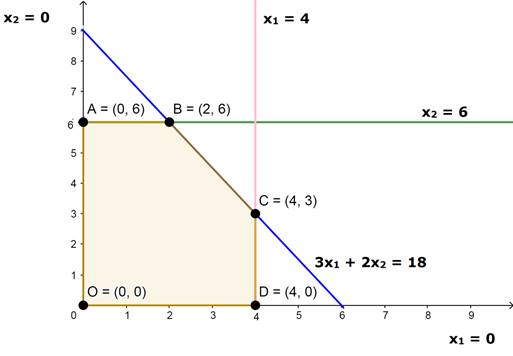
From the graph we can clearly see that the area under the polygon, OABCD is the feasible solution.
For determining the maximum optimum value from the polygon, the points at the vertices of OABCD are substituted in the Z function and see which point will maximize the value of Z = 3x1 + 5x2.

Among the above points, the Z value maximized at point B where x1 = 2 and x2 = 6.
Therefore the solution is, option B.