A couple has 2 children. Find the probability that both are boys if it is known that (i) one of the children is a boy, and (ii) the elder child is a boy.
A couple has two children.
The sample space S = {(B,B),(B,G),(G,B),(G,G)}
Let P(A) be the probability of both being boys.
(i) Let P(B) be the probability of one of them being a boy.
The sample space of B ={(B,B),(B,G),(G,B)}
Let 
be the probability of one of them being a boy and both being boys.
Tip – By conditional probability, 
where 
is the probability of occurrence of the event A given that B has already occurred.
The probability that both are boys given that one of them is a boy:
(ii) Let P(B) be the probability of the elder being a boy.
The sample space of B ={(B,B),(B,G)}
Let 
be the probability of the elder being a boy and both being boys.
Tip – By conditional probability, 
where 
is the probability of occurrence of the event A given that B has already occurred.
The probability that both are boys given that the elder is a boy:
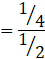
