Given - & . A plane is parallel to both these lines and passes through (3, - 1, 2).

To find – The equation of the plane

Tip – A plane parallel to two vectors will have its normal in a direction perpendicular to both the vectors, which can be evaluated by taking their cross product

& , where the two vectors represent the directions

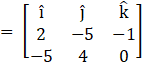
The equation of the plane maybe represented as 4x + 5y - 17z + d = 0

Now, this plane passes through the point (3, - 1, 2)

Hence,

4 × 3 + 5 × (- 1) – 17 × 2 + d = 0

⇒ d = 27

The Cartesian equation of the plane : 4x + 5y - 17z + 27 = 0

The vector equation :