The Cartesian equations of a line are 3x + 1 = 6y – 2 = 1 – z. Find the fixed point through which it passes, its direction ratios and also its vector equation.
Given: Cartesian equation of line are 3x + 1 = 6y – 2 = 1 - z
To find: fixed point through which the line passes through, its direction ratios and the vector equation.
Formula Used: Equation of a line is
Vector form: 
Cartesian form: 
where 
is a point on the line and 
is a vector parallel to the line and also its direction ratio.
Explanation:
The Cartesian form of the line can be rewritten as:
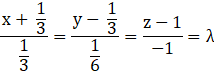
Therefore, 
and 
So, the line passes through 
and direction ratios of the line are (2, 1, -6) and vector form is:
1