Find the Cartesian and vector equations of the line passing through the point (1, 2, -4) and perpendicular to each of the lines 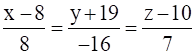and

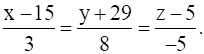
Given: line passes through (1, 2, -4) and is perpendicular to each of the lines and


To find: equation of line in Vector and Cartesian form


Formula Used: Equation of a line is


Vector form:


Cartesian form:


where is a point on the line and is a vector parallel to the line.


If 2 lines of direction ratios a1:a2:a3 and b1:b2:b3 are perpendicular, then a1b1+a2b2+a3b3 = 0


Explanation:


Here,


Let the direction ratios of the line be b1:b2:b3


Direction ratios of other two lines are 8 : -16 : 7 and 3 : 8 : -5


Since the other two line are perpendicular to the given line, we have


8b1 – 16b2 + 7b3 = 0


3b1 + 8b2 – 5b3 = 0


Solving,





Therefore,


Vector form of the line is:



Cartesian form of the line is:



1