Given the boundaries of the area to be found are,

• the circle, x² + y² = 16 ---(1)

• the parabola, x² = 6y ---- (2)

From the equation, of the first circle, x² + y² = 16

• the vertex at (0,0) i.e. the origin

• the radius is 4 unit.

From the equation, parabola, x² = 6y

• the vertex at (0,0) i.e. the origin

• Symmetric about the y-axis, as it has the even power of x.

Now to find the point of intersection of (1) and (2), substitute x² = 6y in (1)

6y + y² = 16

y² + 6y - 16= 0

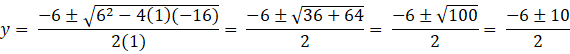
y = 2 (or) y = -8

as x cannot be imaginary, y = 2

Substituting x in (2), we get x = ± 2√3

So the two points, A and B where (1) and (2) meet are A = (2√3,2) and B = (-2√3,2)

As x and y have even powers for both the circle and parabola, they will be symmetrical about the x-axis and y-axis.

Consider the circle, x² + y² = 16, can be re-written as

----- (3)

Consider the parabola, x² = 6y, can be re-written as

----- (4)

Let us drop a perpendicular from A on to x-axis. The base of the perpendicular is D = (2√ 3, 0)

Now, the area to be found will be the area is

Area of the required region = Area of OACBO.

Area of OABCO= Area of OCAO+ Area of OCBO

[area of OCBO= area of OCAOas the circle is symmetrical about the y-axis]

Area of OACBO= 2 × Area of OCAO---- (5)

Area of OCAO= Area of OCAD - Area of OADO

Area of OCAOis

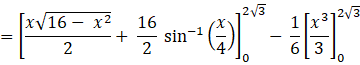
[Using the formula, and ]

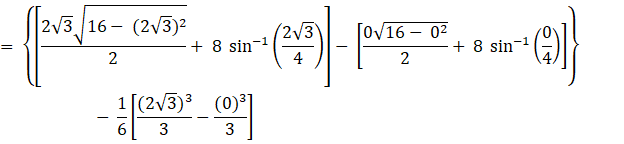
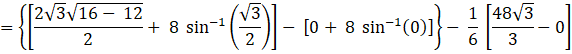
[sin^-1(1) = 90° and ]

The Area of OCAOsq. units

Now substituting the area of OCAOin equation (5)

Area of OACBO= 2 × Area of OCAO

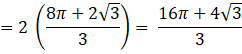
Area of the required region is sq. units.