Given : Equations of lines –

Line 1 :

Line 2 :

To Prove : Line 1 & line 2 are coplanar.

To Find : Equation of plane.

Formulae :

1) Coplanarity of two lines :

If two lines are given by,

and

, then these lines are coplanar, if

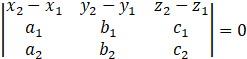
2) Equation of plane :

The equation of plane containing two coplanar lines

& is given by,

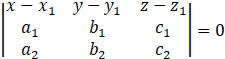
Answer :

Given lines –

Line 1 :

Line 2 :

Here, x₁ = 0 , y₁ = 2 , z₁ = -3 , a₁ = 1 , b₁ = 2 , c₁ = 3

x₂ = 2 , y₂ = 6 , z₂ = 3 , a₂ = 2 , b₂ = 3 , c₂ = 4

Now,

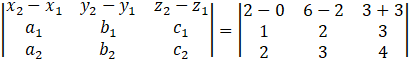
= - 2 + 8 – 6

= 0

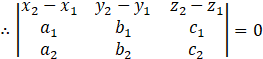
Hence, given two lines are coplanar.

Equation of plane passing through line1 and line 2 is given by,

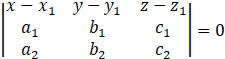
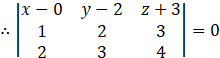
-x + 2y – 4 – z – 3 = 0

- x + 2y – z – 7 = 0

x – 2y + z + 7 = 0

Therefore, equation of plane is

x – 2y + z + 7 = 0