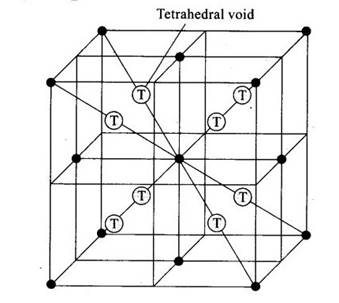
In cubic close packing, each cube consists of eight small cubic components as shown in figure. Since atoms are present in corners and face centres, total atoms are:

Total atoms= atoms at face centres and atoms at corners

==1+3 = 4

Total number of atoms = 4.

Considering single small cubic unit, tetrahedral void in present in body centre. Since here we have cubic structure of 8 small cubic unit, total tetrahedral voids = 8× 1 = 8.

Lets consider single cubic lattice consists of 8 small cubic units, 2 tetrahedral voids are present at each diagonal each equal distance apart from corner. There are 4 diagonal in lattice so total number of tetrahedral voids = 4× 2 =8.

Hence proved. There are 8 tetrahedral voids in CCP.