An ideal gas (CP/CV = γ) is taken through a process in which the pressure and the volume vary as p = αVb. Find the value of b for which the specific heat capacity in the process is zero.

Given:


p = aVb


p = pressure, V = volume, a and b are constants.


Formula used:


We know, Q = U + ഽpdV from first law of thermodynamics,


where Q = change in heat, U = change in internal energy and ഽpdV = W = total work done, p = pressure, V = volume.


Since Q = nCdT, and U = nCvdT, we get


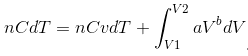… (ii), n = no. of moles, C = specific


heat capacity, and Cv = specific heat capacity at constant volume,


dT = change in temperature,


Since specific heat capacity is 0(given),


…(iii)


(after integration of 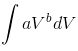from V1 to V2)


Now, from equation of state, PV = nRT,


Where


P = pressure,


V = volume,


n = number of moles,


R = universal gas constant,


T = temperature.


Substituting p = aVb from (i):


aVb+1 = nRT


=> … (iv)


Substituting (iv) in (iii),



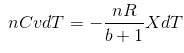(Since (T2 - T1) = dT)


=>


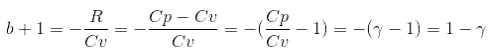
=> b = -

1