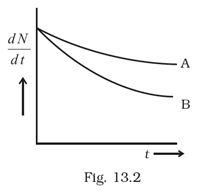
The mean life of B is shorter than that of A

According to the Radioactive Decay Law or Rutherford Soddy Law the decay rate of a nucleus is given by the formula of

,

where

is the rate of decay of the nucleus with respect to time, is the decay rate constant of the nucleus and N is the number of nuclei present in the sample. As shown in the graph the X axis the decay rate and Y axis is the time of decay, now to point the decay rate constant in the graph we have to draw a slope on the curve of sample A and B like this

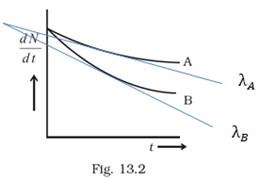
The slope of A and B curve dedicates the decay rate constant of the sample A and B respectively. Now as for the mean life of the samples, the mean life formula is

where

is the mean life and is the decay rate constant. So according to the diagram the mean life of A and B are given as

;

Now as we can see in the above diagram (in the explanation), the value of decay rate constant of B and A is (the flatter the curve the lesser is the slope therefore, the value of the slope of B is greater than A as the curve of B is greater than A) respectively. Hence, due to inverse proportionality the value of mean live of B is lesser than that of A. Therefore, the mean life of B is shorter than that of A.