We know from Gauss Law that the closed surface integral of electric flux () is equal to the charge enclosed () by that closed surface. This is mathematically given by

The electric flux is given by

The charge enclosed by the given Gaussian surface S shown in the figure below is given by

The do not include charge since it is outside the given Gaussian surface.

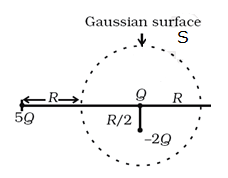
Therefore, using expression (2), the electric flux through the surface of given Gaussian surface is given by

Hence, the electric flux through the given Gaussian surface S is due to charges and only. So, the electric flux through the given Gaussian surface S due to charge is zero.

Therefore, option A and C are correct. The electric field on the given Gaussian surface is due to all charges present in the given figure and s given by