A container contains water up to a height of 20 cm and there is a point source at the center of the bottom of the container. A rubber ring of radius r floats centrally on the water surface. The ceiling of the room is 2.0 m above the water surface. (a) Find the radius of the shadow of the ring formed on the ceiling if r = 15 cm. (b) Find the maximum value of r for which the shadow of the ring is formed on the ceiling. Refractive index of water = 4/3.

Question

Philoid · Accepted Answer

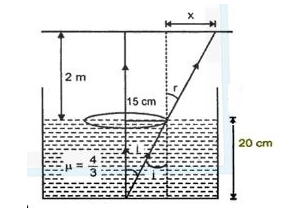
(i)Height of water container h= 20 cm

Ceiling of the room is 2.0 m above the water surface.

Radius of the rubber ring= r

Refractive index of water

From the figure we can say that;

By applying Snell’s law, we get;

⇒

From the figure, we get;

So,

⇒

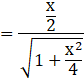
So, the total radius of the shadow =

(b)For Maximum angle of refraction, angle of incidence should be equal to critical angle i.e.

Let R is the maximum radius.

Now,

⇒

⇒ .