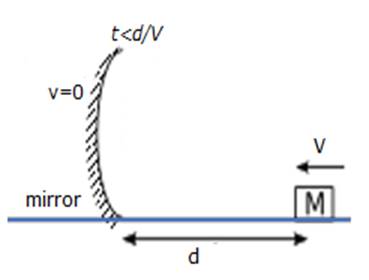
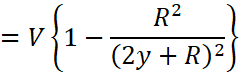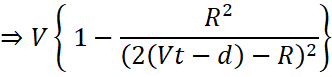A small block of mass m and a concave mirror of radius R fitted with a stand lie on a smooth horizontal table with a separation d between them. The mirror together with its stand has a mass m. The block is pushed at t=0 towards the mirror so that it starts moving towards the mirror at a constant speed V and collides with it. The collision is perfectly elastic. Find the velocity of the image (a) at a time t<d/V, (b) at a time t>d/V.
At time t, the object distance from
mirror, u= -(d-Vt)
Here, t < d/V, and focal length f= -R/2
By using mirror formula,
![]()
![]()
![]()
![]()
Now, differentiating w.r.t ‘t’
![]()
![]()
This is the required speed of mirror.
(b) when t> d/v, the collision between the mirror and mass will take place. Since, the collision is elastic in nature, the object will come to rest and mirror will start to move with velocity V
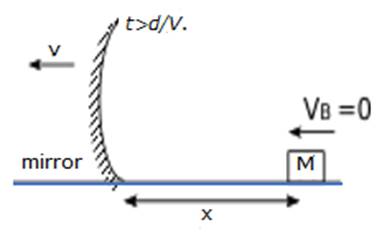
the object distance from mirror, u= (d-Vt)
focal length, f= -R/2
At any time, t > d/v
The distance of mirror from the mass will be
![]()
By using mirror formula,
![]()
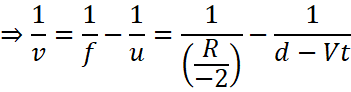
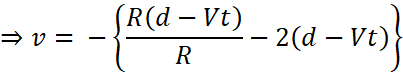
Velocity of the image, V’ is
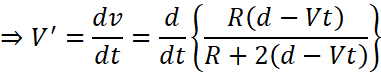
If, y= d-Vt
dy/dt= -V
velocity of the image,
![]()
![]()
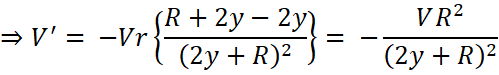
Since, the mirror itself moving with velocity V,
Thus, V= V(image) + V(mirror)
Absolute velocity of image is given by