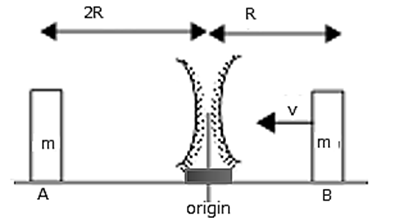
Two concave mirrors of equal radii of curvature R are fixed on a stand facing opposite directions. The whole system has a mass m and is kept on a frictionless horizontal table (figure 18-E15).

Two blocks A and B, each of mass m, are placed on the two sides of the stand. At t = 0, the separation between A and the mirrors is 2R and also the separation between B and the mirrors is 2R. The block B moves towards the mirror at a speed v. All collisions which take place are elastic. Taking the original position of the mirrors-stand system to be x = 0 and X-axis along AB, find the position of the images of A and B at t =
![]()
(a) ![]()
(b) ![]()
(c) ![]()
Mass of the system= M
Mass of the two blocks A and B= m
Radii of curvature of two concave mirror= R
At time t=0
Distance between two block A and B= 2R
Block B is moving at a speed v towards mirror.
Original position of whole system, x=0
(a) At time t= R/v
Distance moved by block B towards mirror= v x R/v= R
The distance of Block A from mirror=, u=-R
Focal length of the mirror, f= -R/2
By using mirror formula,
![]()
![]()
![]()
For the block A,
The object distance, u= -2R
By using mirror formula,
![]()
![]()
![]()
So, with respect to the given coordinate system, Position of A and B are ![]() respectively from origin.
respectively from origin.
(b) At time t= 3R/v
The block B after colliding with mirror must have come to rest because it is an elastic collision and the mirror have travelled a distance R towards left form its initial position.
So, at this point of time, for block A,
The object distance, u= -R

By using mirror formula,
![]()
![]()
![]()
Therefore, the position of the image of block A is at -2R with respect to the given coordinate system. For block B, the image of block b is at the same place as it is at a distance of r from the mirror. Therefore, the image of the block B is zero with respect to the given coordinate system. Positions of images of A and B are = –2R, 0 from origin.
(c) at time t= 5R/v
in the similar way, it can be proved that the position of the image of block A and B will be -3R and -4R/3 respectively
