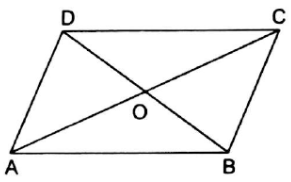
In the adjoining figure, O is any point inside a parallelogram ABCD. Prove that
(i) ar(∆OAB) + ar(∆OCD) = ![]() ar(‖gm ABCD),
ar(‖gm ABCD),
(ii)ar(∆OAD) + ar(∆OBC) = ![]() ar(‖gm ABCD).
ar(‖gm ABCD).
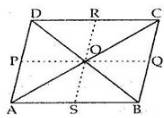
Given : A parallelogram ABCD with a point ‘O’ inside it.
To prove : (i) area(∆OAB) + area(∆OCD) = ![]() area(‖gm ABCD),
area(‖gm ABCD),
(ii)area(∆OAD) + area(∆OBC) = ![]() area(‖gm ABCD).
area(‖gm ABCD).
Construction : Draw PQ ‖ AB and RS ‖ AD
Proof:
(i)
∆AOB and parallelogram ABQP have same base AB and lie between parallel lines AB and PQ.
According to theorem: If a triangle and parallelogram are on the same base and between the same
parallel lines, then the area of the triangle is equal to half of the area of the parallelogram.
![]() area(∆AOB) =
area(∆AOB) = ![]() area(‖gm ABQP) –--1
area(‖gm ABQP) –--1
Similarly, we can prove that area(∆COD) = ![]() area(‖gm PQCD) –--2
area(‖gm PQCD) –--2
![]() Adding –1 and –2 we get,
Adding –1 and –2 we get,
area(∆AOB) + area(∆COD) = ![]() area(‖gm ABQP) +
area(‖gm ABQP) + ![]() area(‖gm PQCD)
area(‖gm PQCD)
area(∆AOB) + area(∆COD) = ![]()
![]() =
= ![]() area(‖gm ABCD)
area(‖gm ABCD)
![]() area(∆AOB) + area(∆COD) =
area(∆AOB) + area(∆COD) = ![]() area(‖gm ABCD)
area(‖gm ABCD)
Hence proved
(ii)
∆OAD and parallelogram ASRD have same base AD and lie between parallel lines AD and RS.
According to theorem: If a triangle and parallelogram are on the same base and between the same
parallel lines, then the area of the triangle is equal to half of the area of the parallelogram.
![]() area(∆OAD) =
area(∆OAD) = ![]() area(‖gm ASRD) –--1
area(‖gm ASRD) –--1
Similarly, we can prove that area(∆OBC) = ![]() area(‖gm BCRS) –--2
area(‖gm BCRS) –--2
![]() Adding –1 and –2 we get,
Adding –1 and –2 we get,
area(∆OAD) + area(∆OBC) = ![]() area(‖gm ASRD) +
area(‖gm ASRD) + ![]() area(‖gm BCRS)
area(‖gm BCRS)
area(∆OAD) + area(∆OBC) = ![]()
![]() =
= ![]() area(‖gm ABCD)
area(‖gm ABCD)
![]() area(∆OAD) + area(∆OBC) =
area(∆OAD) + area(∆OBC) = ![]() area(‖gm ABCD)
area(‖gm ABCD)
Hence proved