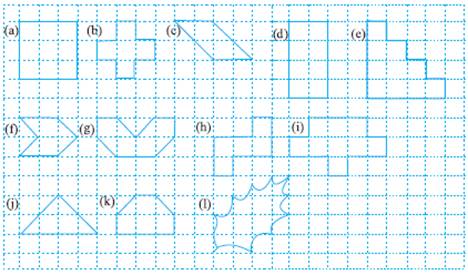
(a) By observing the figure, we see that it contains 9 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 9 square units.

(b) By observing the figure, we see that it contains 5 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 5 square units.

(c) By observing the figure, we see that it contains 2 fully filled squares and 4 half- filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 2 + (4× 0.5) = 2+ 2 = 4 square units.

(d) By observing the figure, we see that it contains 8 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 8 square units.

(e) By observing the figure, we see that it contains 10 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 10 square units.

(f) By observing the figure, we see that it contains 2 fully filled squares and 4 half- filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 2 + (4× 0.5) = 2+ 2 = 4 square units.

(g) By observing the figure, we see that it contains 4 fully filled squares and 4 half- filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 4 + (4× 0.5) = 4 + 2 = 6 square units.

(h) By observing the figure, we see that it contains 5 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 5 square units.

(i) By observing the figure, we see that it contains 9 fully filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 9 square units.

(j) By observing the figure, we see that it contains 2 fully filled squares and 4 half- filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 2 + (4× 0.5) = 2+ 2 = 4 square units.

(k) By observing the figure, we see that it contains 4 fully filled squares and 2 half- filled squares.

If the area of one such square is taken to be as 1 square unit.

Then,

The area of the figure = 4 + (2× 0.5) = 4 + 1 = 5 square units.

(l) In the figure given, we find that there are variations in the filling of the squares.

Thus, we form a table to organise the data by observing the above figure.

Therefore,

Total Area = 2 + 6 = 8 square units

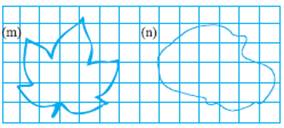
(m) In the figure given, we find that there are variations in the filling of the squares.

Thus, we form a table to organise the data by observing the above figure.

Therefore,

Total Area = 5 + 9 = 14 square units

(n) By observing above figure, we get

Therefore,

Total Area = 8 + 10 = 18 square units