The perimeter of a rhombus is 180 cm and one of its diagonals is 72 cm. Find the length of the other diagonal and the area of the rhombus.

Question

Philoid · Accepted Answer

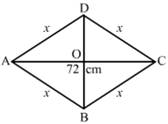
Given: A rhombus

Diagonal AC = 72 cm

Perimeter = 180 cm

Perimeter of the rhombus = 4x

Therefore 4x = 180

x= 45

hence, the side length of the rhombus is 45 cm

We know that diagonals of the rhombus bisect each other right angles.

AO = AC

⇒AO = ( × 72) cm

⇒AO = 36 cm

From right AOB, we have :

BO² = AB² – AO²

⇒BO² = AB² – AO²

⇒BO² = 45² – 36²

⇒BO² = 2025 – 1296

⇒BO² = 729

BO = 27 cm

BD = 2× BO

BD = 2 × 27 = 54 cm

Hence, the length of the other diagonal is 54 cm.

Area of the rhombus = × 72 × 54 = 1944 cm²