(i) From the given figure, we have

y + 120^o = 180^o (Linear pair)

Therefore,

y = 180^o – 120^o

y = 60^o

x + y + 50^o = 180^o (angle sum property of triangle)

x + 110^o = 180^o

x = 180^o – 110^o

x = 70^o

Hence,

The value of x = 70^o

And,

Value of y = 60^o

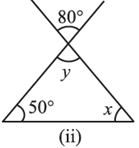
(ii) From the given figure, we have

y = 80^o (Vertically opposite angle)

y + x + 50^o = 180^o (angle sum property of triangle)

80^o + x + 50^o = 180^o

130^o + x = 180^o

x = 180^o – 130^o

x = 50^o

Hence,

The value of x = 50^o

And,

The value of y = 80^o

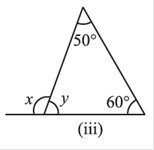
(iii) From the given figure,

y + 50^o + 60^o = 180^o (angle sum property of triangle)

y + 110^o = 180^o

y = 180^o – 110^o

y = 70^o

x and y are on a straight line and forming a linear pair

Therefore,

x + y = 180^o

x + 70^o = 180^o

x = 180^o – 70^o

x = 110^o

Hence,

The value of x = 110^o

And,

Value of y = 70^o

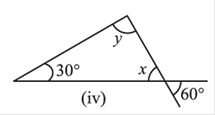
(iv) From the given figure, we have

x = 60^o (Vertically opposite angle)

30^o + x + y = 180^o (angle sum property of triangle)

30^o + 60^o + y = 180^o

90^o + y = 180^o

y = 180^o – 90^o

y = 90^o

Hence,

The value of x = 60^o

And,

Value of y = 90^o

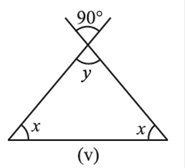
(v) From the given figure, we have

y = 90^o (Vertically opposite angle)

x + x + y = 180^o (angle sum property of triangle)

2x + y = 180^o

2x + 90^o = 180^o

2x = 180^o – 90^o

2x = 90^o

x =

x = 45^o

Hence, The value of x = 45^o

And,

The valuue of y = 90^o

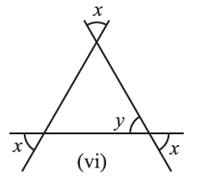
(vi) From the given figure, we have

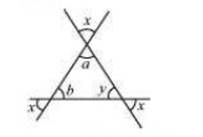
y = x (Vertically opposite angles)

a = x (Vertically opposite angles)

b = x (Vertically opposite angles)

a + b + x = 180^o (angle sum property of triangle)

x + x + x = 180^o

3x = 180^o

x =

x = 60^o

y = x (Vertically opposite angle)

Hence,

The value of y = x = 60^o