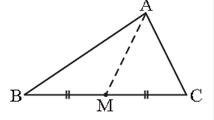
AM is a median of a triangle ABC. Is AB + BC + CA > 2AM? (Consider the sides of triangles ABM and AMC)
We know that,
In a triangle,
The sum of the length of either two sides of the triangle is always greater than the third side
In the given question, we have to show that:
AB + BC + CA > 2 AM
Therefore,
In ![]() ABM, we have:
ABM, we have:
AB + CM > AM (i)
Same is the case with ![]() ACM, in which:
ACM, in which:
AC + CM > AM (ii)
Adding (i) and (ii), we get
AB + BM + MC + AC > AM + AM
AB + BC + AC > 2 AM
44