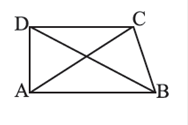
It is given in the question that,

A quadrilateral ABCD, in which we have to show that:

Whether AB + BC + CD + DA > AC + BD or not

We know that,

In a triangle,

The sum of the length of either two sides of the triangle is always greater than the third side

Therefore,

In ∆ABC, we have

AB + BC > CA (i)

In ∆BCD, we have

BC + CD > DB (ii)

In ∆CDA, we have

CD + DA > AC (iii)

In ∆DAB, we have

DA + AB > DB (iv)

Adding equations (i), (ii), (iii) and (iv), we get:

AB + BC + BC + CD + CD + DA + DA + AB > AC + BD + AC + BD

2 AB + 2 BC + 2 CD + 2 DA > 2 AC + 2 BD

2 (AB + BC + CD + DA) > 2 (AC + BD)

(AB + BC + CD + DA) > (AC + BD)

Hence, the expression given in the question is true