Find the values of the angles x, y and z in each of the following:
(i) 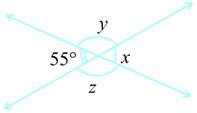
(ii) 
(i) We have to find the value of x, y and z
We have,
∠x and 55o are vertically opposite angles
Therefore,
∠x = 55o
Also,
∠x and ∠y form a linear pair
Therefore,
∠x + ∠y = 180o (Sum of linear pair angles)
55o + ∠y = 180o
∠y = 180o – 55o
= 125o
Also,
∠y and ∠z are vertically opposite angles
Therefore,
∠y = ∠z = 125o
Hence,
The value of x, y and z is as follows:
∠x = 55o
∠y = 125o
And,
∠z = 125o
(ii) We have to find out the values of x, y and z
We have,
∠z and 40o are vertically opposite angles
Therefore,
∠z = 40o
Also,
∠y and ∠z form a linear pair
Therefore,
∠y + ∠z = 180o (Sum of angles of linear pair)
∠y + 40o = 180o
∠y = 180o – 40o
= 140o
Also, we know that:
Sum of angles in a straight line = 180o
∠x + 40o + 25o = 180o
∠x + 40o + 25o = 180o
∠x + 65o = 180o
∠x = 180o – 65o
= 115o
Hence,
The value of ∠x, ∠y and ∠z is as follows:
∠x = 115o
∠y = 140o
∠z = 40o