In the figures given below, decide whether l is parallel to m.
(i) 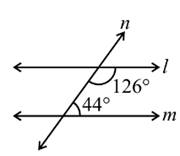
(ii) 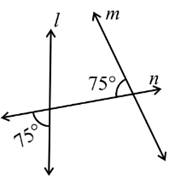
(iii) 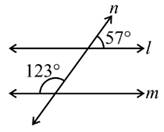
(iv) 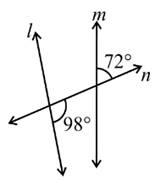
(i) From the above figure,
There are two angles 126o and 44o which are on the same side of the transversal line n
Let us consider two lines l and m
Also,
There is a transversal line n which is intersecting both the lines
Therefore,
Sum of interior angles on the same side of the transversal = 126o + 44o
= 170o
Hence,
It is clear that the sum of interior angles which are on the same side of the transversal is not equal to 180o
Therefore,
The lines l and m are not parallel to each other
(ii) In the above question we have, Angle x and 75o are forming a linear pair on the line l

Therefore,
x + 75o = 180o (Sum of angles of linear pair)
x = 180o – 75o
= 105o
We have to show that l and m are parallel to each other
For this,
Corresponding angles ∠ABC and ∠x should be equal
But,
∠x = 105o
And,
∠ABC = 105o
Therefore,
Lines l and m are not parallel to each other
(iii) In the above question we have, Angle x and 123o are forming a linear pair on the line m

Therefore,
x + 123o = 180o (Sum of angles of linear pair)
x = 180o – 123o
= 57o
We have to show that l and m are parallel to each other
For this,
Corresponding angles ∠ABC and ∠x should be equal
∠x = 57o
And,
∠ABC = 57o
Therefore,
Both the angles are equal to each other
Hence,
Lines l and m are not parallel to each other
(iv) In the above question we have, Angle x and 98o are forming a linear pair on the line l

Therefore,
x + 98o = 180o (Sum of angles of linear pair)
x = 180o – 98o
= 82o
We have to show that l and m are parallel to each other
For this,
Corresponding angles ∠ABC and ∠x should be equal
But,
∠x = 82o
And,
∠ABC = 72o
Therefore,
Lines l and m are not parallel to each other