In the given figure, it is given that AD=BC and AC=BD. Prove that ∠ CAD= ∠ CBD and ∠ ADC= ∠ BCD.

Question

Philoid · Accepted Answer

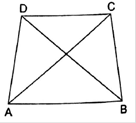
From the given figure,

In triangles DAC and CBD, we have:

AD = BC

AC = BD

DC = DC

So, by SSS congruence rule

∆ADC ≅ ∆BCD

∴ By Congruent parts of congruent triangles we have:

∠CAD = ∠CBD

∠ADC = ∠BCD

∠ACD = ∠BDC

Hence, proved