Let P(x) = 6x³ + 2x² - 10x - 42

As √2 is one of the zeroes of P(x).

⇒ g(x) = (x - √2) is one of the factors of P(x).

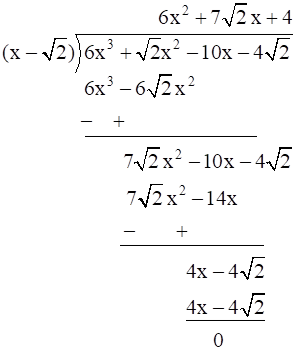
By division algorithm

Dividend = (divisor) (quotient) + remainder

i.e. p(x) = g(x)q(x) + r(x)

clearly r(x) = 0 and q(x) = 6x² + 7√2 + 4

⇒ 6x³ + √2x² - 10x - 4√2 = (x - √2) (6x² + 7√2 + 4)

6x³ + √2x² - 10x - 4√2 = 0

= (x - √2) (6x² + 7√2 + 4) = 0

= (x - √2) {6x² + (3√2x + 4√2x) + 4} = 0 (by splitting the middle term)

= (x - √2) {6x² + 3√2x + 4√2x + 4} = 0

= (x - √2) {3√2x (√2x + 1) + 4(√2x + 1)} = 0

= (x - √2) (√2x + 1) (3√2x + 4) = 0

⇒ x = - 1/√2, 2√2/3, √2