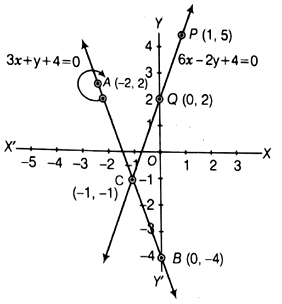
By the graphical method, find whether the following pair of equations are consistent or not. If consistent, solve them.
3x + y + 4 = 0, 6x-2y + 4 = 0
Given pair of equations is
3x + y + 4 = 0 …(i)
and 6x -2y + 4 = 0…(ii)
comparing with ax + by + c = 0
Here, a1 = ![]() , b1 = 1, c1 = 4;
, b1 = 1, c1 = 4;
And a2 = 6, b2 = - 2, c2 = 4;
a1 /a2 = 1/2
b1 /b2 = -3/6 = -1/2
c1 /c2 = 1
since a1/a2 ![]() b1/b2
b1/b2
so system of equations is consistent with a unique solution.
We have, ![]()
![]()
When x = 0, then y = - 4
When x = - 1, then y = - 1
When x = - 2, then y = 2
| 0 | - 1 | - 2 |
y | - 4 | - 1 | 2 |
Points | B | C | A |
and ![]()
![]()
![]()
When x = 0, then y = 2
When x = - 1,then y = - 1
When x = 1,then y = 5
| - 1 | 0 | 1 |
y | - 1 | 2 | 5 |
Points | C | Q | P |
Plotting the points B(0, - 4) and A( - 2,2),we get the straight tine AB. Plotting the points Q(0,2) and P(1,5) we get the straight line PQ. The lines AB and PQ intersect at C (-1, -1).