The sum of the first n terms of an AP whose first term is 8 and the common difference is 20 is equal to the sum of first 2n terms of another AP whose first term is - 30 and the common difference is 8. Find n.

Question

Philoid · Accepted Answer

Given that, first term of the first AP, a = 8 and that of second AP, A = - 30

and common difference of the first AP, d = 20 and that of second AP, D = 8

Given that

Sum of first n terms of first AP = Sum of first 2n terms of second AP

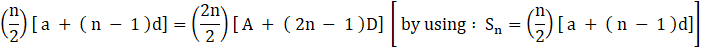
a + (n - 1)d = 2[ A + (2n - 1)D]

8 + (n - 1)20 = 2[ - 30 + (2n - 1)8]

8 + 20n - 20 = 2[ - 30 + 16n - 8]

8 + 20n - 20 = - 60 + 32n - 16

20n - 16 = 32n - 76

32n - 20n = 76 - 16

12n = 60

n = 5