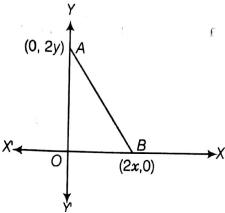
The coordinates of the point which is equidistant from the three vertices of the ΔAOB as shown in the figure is
![]()
Let the coordinate of the point P is (h, k).
Given,
Point P is equidistant from the three vertices O, A and B
Where;
O (0, 0),
A (0, 2y) and
B (2x, 0)
Then,
PO = PA = PB
→ (PO)2 = (PA)2 = (PB)2 ….(i)
By using distance formula,
![]()
= ![]()
= ![]()
= h2 + k2 = h2 + (k – 2y)2 …..(ii)
= (h – 2x)2 + k2 ….. (iii)
Taking first two equations, we get
h2 + k2 = h2 + (k – 2y)2
→ k2 = k2 + 4y2 – 4yk → 4y(y – k) = 0
→ y = k [∵ y ≠ 0]
Taking first and third equations, we get
h2 + k2 = (h – 2x)2 + k2
→ h2 + h2 + 4x2 – 4xh
→ 4x (x – h) = 0
→ x = h [∵ x ≠ 0]
∴ Required points = (h, k) = (x, y)
15