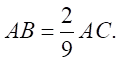
True

We know by the rule that, area of a triangle formed by the points (x₁, y₁), (x₂, y₂) and (x₃, y₃) is zero, then the points are collinear.

Area of the triangle = 1/2 [x₁ (y₂ - y₃ ) + x₂ (y₃ - y₁ ) + x₃ (y₁ - y₂ )]

Here we have;

x₁ = - 6

x₂ = - 4

x₃ = 3

and

y₁ = 10

y₂ = 6

y₃ = - 8

Area of the triangle ∆ ABC = 1/2 [ - 6 {6 – ( - 8)} + ( - 4) ( - 8 – 10) + 3(10 – 6)]

= 1/2 [ - 6(14) + ( - 4) ( - 18) + 3(4)]

= 1/2 ( - 84 + 72 + 12) = 0

So, given points are collinear.

Now,

Calculate the distance between A ( - 6, 10) and B ( - 4, 6);

∵ Distance between the points (x₁, y₁) and (x₂, y₂);

Distance between A ( - 6, 10) and C (3, - 8);

∴ AB=2/9 AC