Find the ratio in which the point 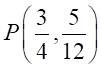 divides the line segment joining the points
divides the line segment joining the points 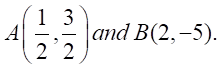
Let P ![]() divide AB internally in the ratio m:n.
divide AB internally in the ratio m:n.
Using the section formula,
Internal section formula, the coordinates of point P divides the line segment joining the point (x1, y1), (x2, y2) in the ratio m1:m2 internally is
![]()
We get;
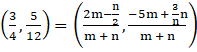
On equating,
We get;
![]()
![]()
![]()
And
![]()
![]()
![]()
3m + 3n = 8m – 2n
5n – 5m = 0
n = m
And, 5m + 5n = - 60 + 18n
65m – 13n = 0
13(5m – n) = 0
5m – n = 0
Since,
m = n does not satisfy.
5m - n = 0
5m = n
![]()
Hence, the required ratio is 1:5.
12