If  and
and 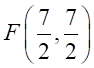 are the mid - points of sides of ΔABC, then find the area of the ΔABC.
are the mid - points of sides of ΔABC, then find the area of the ΔABC.
Let the vertices of ∆ABC are;
A = (x1, y1)
B = (x2, y2)
C = (x3, y3)
Given,
D, E and F are the mid - points of the sides BC, CA and AB respectively.
Where Coordinates are;
D = ![]()
E = (7, 3)
F = ![]()
By mid - point formula of a line segment having points (x1, y1) and (x2, y2) = ![]()
So,
D![]() is the mid - point of BC
is the mid - point of BC
∴ ![]()
And
![]()
![]() x2 + x3 = - 1 …..(i)
x2 + x3 = - 1 …..(i)
And
y2 + y3 = 5 … (ii)
As E (7, 3) is the mid - point of CA
∴ ![]()
And
![]()
∴ x3 + x1 = 14 .. (iii)
And
Y3 + y1 = 6 …. (iv)
Also,
F![]() is the mid - point of AB
is the mid - point of AB
∴ ![]()
And
![]()
∴ x1 + x2 = 7 … (v)
And
y1 + y2 = 7 …(vi)
On adding equations (i), (iii) and (v),
We get,
2(x1 + x2 + x3) = 20
x1 + x2 + x3 = 10………(vii)
On subtracting Equations (i), (iii) and (v) from Eq. (vii) respectively,
We get;
x1 = 11,
x2 = - 4
x3 = 3
On adding Equations (ii), (iv) and (vi),
We get;
2(y1 + y2 + y3) = 18
y1 + y2 + y3 = 9…..(viii)
On subtracting Equations (ii), (iv) and (vi) from Eq. (viii) respectively,
We get;
y1 = 4
y2 = 3
y3 = 2
Hence, the vertices of ΔABC are A (11, 4), B ( - 4, 3) and C (3, 2)
So,
∵ Area of ∆ABC = 1/2 [x1 (y2 – y3 ) + x2 (y3 - y1 ) + x3 (y1 - y2 )]
∆ = ![]() [11(3 – 2) + ( - 4)(2 – 4) + (4 – 3)]
[11(3 – 2) + ( - 4)(2 – 4) + (4 – 3)]
= ![]() [11 × 1 + ( - 4)( - 2) + 3(1)]
[11 × 1 + ( - 4)( - 2) + 3(1)]
= ![]() [11 + 8 + 3] = 22/2 = 11
[11 + 8 + 3] = 22/2 = 11
∴ Required area of ΔABC = 11