The value of 2 sin ![]() can be a +
can be a +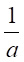 , where a is a positive number and a
, where a is a positive number and a![]() 1.
1.
False
Given: ‘a’ is a positive number and a≠1
⇒ AM > GM
(Arithmetic Mean (AM) of a list of non- negative real numbers is greater than or equal to the Geometric mean (GM) of the same list)
If a and b be such numbers, then
![]() and GM = √ab
and GM = √ab
By assuming that ![]() statement is be true.
statement is be true.
Similarly, AM and GM of a and 1/a are (a+1/a)/2 and √(a.1/a) respectively.
By property, (a+1/a)/2 > √(a.1/a)
![]()
⇒ 2 sin θ > 2 (By our assumption)
⇒ sin θ > 1
But -1 ≤ sin θ ≤ 1
∴ Our assumption is wrong and that 2 sin θ cannot be equal to ![]()
9