The angle of elevation of the tower from certain point is 300. If the observer moves 20 m towards the tower, the angle of elevation of the top increase by 150.Find the height of the tower.
Let PR = h meter, be the height of the tower.
The observer is standing at point Q such that, the distance between the observer and tower is QR = (20+x) m, where
QR = QS + SR = 20 + x
∠PQR = 30°
∠ PSR = θ
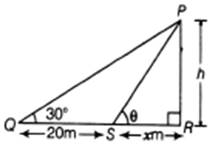
In ∆PQR,
![]()
![]()
Rearranging the terms,
We get 20 +x = √3 h
![]()
In ∆PSR,
![]()
Since, angle of elevation increases by 15![]() when the observer moves 20 m towards the tower. We have,
when the observer moves 20 m towards the tower. We have,
θ = 30° + 15° = 45°
So,
![]()
![]()
⇒ h = x
Substituting x=h in eq. 1, we get
h = √3 h – 20
⇒ √3 h – h = 20
⇒ h (√3 - 1) = 20
![]()
Rationalizing the denominator, we have
![]()
![]()
![]()
![]()
= 10 (√3 + 1)
Hence, the required height of the tower is 10 (√3 + 1) meter.