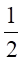Given: 1+sin² θ = 3 sin θ cos θ

Dividing L.H.S and R.H.S equations with sin² θ,

We get

⇒ cot² θ +1+1 = 3 cot θ [∵, cosec² θ – cot² θ = 1 ⇒ cosec² θ = cot² θ +1]

⇒ cot² θ +2 = 3 cot θ

⇒ cot² θ –3 cot θ +2 = 0

Splitting the middle term and then solving the equation,

⇒ cot² θ – cot θ –2 cot θ +2 = 0

⇒ cot θ(cot θ -1)–2(cot θ +1) = 0

⇒ (cot θ - 1)(cot θ - 2) = 0

⇒ cot θ = 1, 2

Hence, proved.