The angle of elevation of the top of a tower from two points distant s and t from its foot are complementary. Prove that the height of the tower is .

Question

Philoid · Accepted Answer

Let BC = s; PC = t

Let height of the tower be AB = h.

∠ABC = θ and ∠APC = 90° - θ

(∵ the angle of elevation of the top of the tower from two points P and B are complementary)

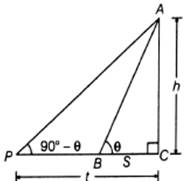
In

Multiplying eq. 1 and eq. 2, we get

⇒ h² = st

⇒ h = √st

Hence the height of the tower is √st.