The shadow of a tower standing on a level plane is found to be 50m longer when sun’s elevation is 30 0 than when it is 60 0 . Find the height the tower.

Question

Philoid · Accepted Answer

Let SQ = h be the tower.

∠SPQ = 30° and ∠SRQ = 60°

According to the question, the length of shadow is 50 m long hen angle of elevation of the sun is 30° than when it was 60°. So,

PR = 50 m and RQ = x m

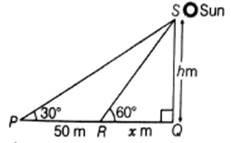
So in ∆SRQ, we have

In ∆SPQ,

Substituting the value of x in the above equation, we get

⇒ 50√3+h = 3h

⇒ 50√3 = 3h - h

⇒ 3h - h = 50√3

⇒ 2h = 50√3

⇒ h = (50√3)/2

⇒ h = 25√3

Hence, the required height is 25√3 m.

The shadow of a tower standing on a level plane is found to be 50m longer when sun’s elevation is 300 than when it is 600. Find the height the tower.

The shadow of a tower standing on a level plane is found to be 50m longer when sun’s elevation is 30⁰ than when it is 60⁰. Find the height the tower.