A vertical tower stands on a horizontal plane and is surmounted by a vertical flag staff of height h. At a point on the plane, the angles of elevation of the bottom and the top of the flag staff are α and β respectively. Prove that the height of the tower is  .
.
Given that a vertical flag staff of height h is surmounted on a vertical tower of height H(say),
such that FP = h and FO = H.
The angle of elevation of the bottom and top of the flag staff on the plane is ∠PRO = α and ∠FRO = β respectively.
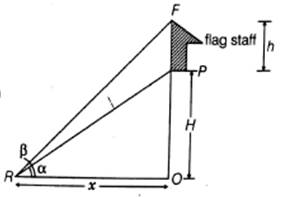
In ∆PRO, we have
![]()
![]()
![]()
And in ∆FRO, we have
![]()
![]()
Comparing eq. 1 and eq. 2,
![]()
Solving for H,
⇒ H tan β = (h+H) tan α
⇒ H tan β – H tan α = h tan α
⇒ H (tan β – tan α) = h tan α
![]()
Hence, proved.
8