In the given figure, O is a centre of a circle. If ∠OAB = 40° and C is a point on the circle, then ∠ACB = ?

Question

In the given figure, O is a centre of a circle. If ∠ OAB = 40° and C is a point on the circle, then ∠ ACB = ?

Philoid · Accepted Answer

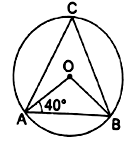
In Δ AOB OA = OB( radius)

OAB = OBA (Angles opposite to equal sides are equal)

OBA = 40

By angle sum property

OAB + OBA + AOB = 180°

AOB = 180° – OAB – OBA

AOB = 180° – 40° – 40° = 100°

We know that

2 ×ACB = AOB (The angle subtended by an arc at the center is twice the angle subtended by the same arc on any point on the remaining part of the circle).

2 ×ACB = 100°

ACB =

ACB = 50