Match the following columns:
Column I | Column II |
(a) Angle in a semicircle measures | (p) 40o |
(b) In the given figure, O is the centre of a circle. If ∠AOB = 120°, then ∠ACB = ?
| (q) 80o |
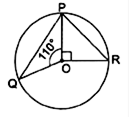
(c) In the given figure, O is the centre of a circle. If ∠POR = 90° and ∠POQ = 110°, then ∠QPR = ?
| (r) 90o |
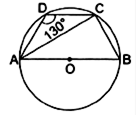
(d) In cyclic quadrilateral ABCD, it is given that ∠ADC = 130° and AOB is a diameter of the circle through A, B, C and D. Then, ∠BAC = ?
| (s) 60o |
The correct answer is:
(a) – ……, (b) – ………,
(c) – …….., (d) – ………,
(a) Angle in a semicircle measures – 90° (r)
(b) In the given figure, O is the centre of a circle. If ∠AOB = 120°, then ∠ACB = ?
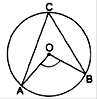
![]() ∠AOB = ∠ACB
∠AOB = ∠ACB
∠ACB = ![]() × 120° = 60°
× 120° = 60°
∠ACB = 60° (s)
(c) In the given figure, O is the centre of a circle. If ![]() and
and ![]() then
then ![]()
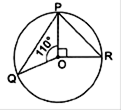
Here, OP = OR = OQ (radius)
In ΔPOR
∠OPR = ∠ORP (angles opposite to equal sides are equal)
By angle sum property
∠POR + ∠OPR + ∠ORP = 180°
90° + 2×∠OPR = 180°
2×∠OPR = 180°—90°
2×∠OPR = 90°
∠OPR = 45°
Similarly in Δ POQ
∠OPQ = ∠OQP (angles opposite to equal sides are equal)
By angle sum property
∠POQ + ∠OPQ + ∠OQP = 180°
110° + 2×∠OQP = 180°
2×∠OQP = 180°–110°
2×∠OQP = 70°
∠OQP = 35°
∠QPR = ∠QPO + ∠OPR = 45° + 35° = 80°
∴ ∠QPR = 80° (q)
(d) In cyclic quadrilateral ![]() it is given that
it is given that ![]() and
and ![]() is a diameter of the circle
is a diameter of the circle
through ![]() and
and ![]() Then,
Then, ![]()

Here,
∠ADC + ∠ABC = 180° (opposite angles in cyclic quadrilateral are supplymentary)
130° + ∠ABC = 180°
∠ABC = 180°–130° = 50°
In ΔABC
By angle sum property
∠BAC + ∠ABC + ∠ACB = 180°
∠BAC + 50° + 90° = 180°
∠BAC = 180°–50°–90° = 40°
∴∠BAC = 40° (p)
∴ Answers are: (a) – (r), (b) – (s), (c) – (q), (d) – (p)